从三维三角形曲面网格中提取域和区域的快速算法
IF 3.1
3区 计算机科学
Q2 COMPUTER SCIENCE, SOFTWARE ENGINEERING
引用次数: 0
摘要
正确的网格处理,特别是域提取,是许多仿真工作流程的重要步骤。例如,在边界元方法中,只有任意形状的域的表面需要网格化。这里介绍一种算法,它可以从给定的非平面三维曲面三角剖分中提取所有不同的空间区域和域,而无需体积网格。只需给出网格顶点的坐标和三角形的连接矩阵。该过程稳健、易于实现且高效。该方法无需检测或指定边或面等几何特征。因此,该方法适用于不包含几何分割信息的网格格式。本文详细介绍了该算法,讨论了测试案例,并给出了示例实现方法。本文章由计算机程序翻译,如有差异,请以英文原文为准。
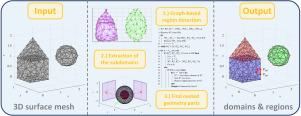
Fast algorithm for extracting domains and regions from three-dimensional triangular surface meshes
Correct mesh handling and in particular domain extraction is an important step of many simulation workflows. In the boundary element method, for example, only the surfaces of arbitrarily shaped domains have to be meshed. Here, an algorithm is presented that extracts all distinct spatial regions and domains from a given non-manifold three-dimensional surface triangulation without the need for a volume mesh. Only the coordinates of the mesh vertices and the connectivity matrix of the triangulation need to be given. The process is robust, easy to implement, and efficient. No geometric features such as edges or faces need to be detected or specified for the method to work. Thus, the method is suitable for mesh formats that do not contain information about the geometry partitioning. The algorithm is presented in detail, test cases are discussed, and an exemplary implementation is given.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Computer-Aided Design
工程技术-计算机:软件工程
CiteScore
5.50
自引率
4.70%
发文量
117
审稿时长
4.2 months
期刊介绍:
Computer-Aided Design is a leading international journal that provides academia and industry with key papers on research and developments in the application of computers to design.
Computer-Aided Design invites papers reporting new research, as well as novel or particularly significant applications, within a wide range of topics, spanning all stages of design process from concept creation to manufacture and beyond.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


