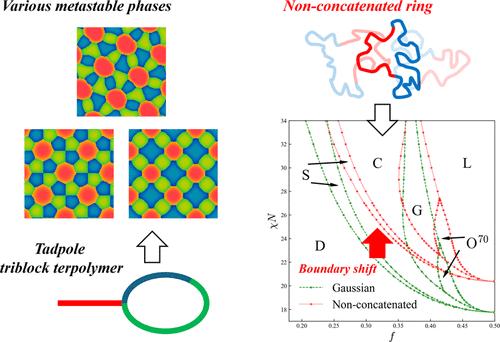
Density Functional Theory for Cyclic Block Copolymer Melts

We propose an efficient method for the self-assembly of Gaussian block copolymers with general cyclic architectures and nonconcatenated ring block copolymer in a melt based on a Ginzburg–Landau-type density functional theory combined with random phase approximation. For the Gaussian copolymers, the applicability of the density functional theory is enhanced by a Gaussian embedding method with a graph Laplacian, which allows evaluating single-chain scattering functions for arbitrary architectures including internal multicycles without analytical difficulty. By using this methodology, we predict phase diagrams of ring and bicycle diblock copolymers at the same cost as a linear diblock copolymer, and discover various metastable morphologies of a tadpole triblock terpolymer, which have not been observed for linear and star triblock terpolymers. We also demonstrate that our framework predicts the phase diagram of the nonconcatenated ring diblock copolymer with the aid of its single-chain scattering function obtained by experiments.