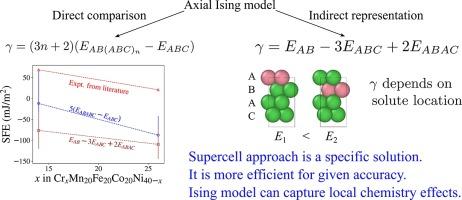
Exploring stacking fault energy with the axial Ising model: A renewed approach

In this work we revisit the axial Ising model for computing intrinsic stacking fault energies (${\gamma }_{{}_{ISF}}$), which are important in alloy design. We find that the supercell approach, which directly compares the energies of fcc stacking with and without a fault, is a specific solution of the Ising model and is the most elegant and efficient among the solutions of the same order of accuracy. Contrary to the traditional belief that only the supercell approach can capture the effect of local chemistry, we demonstrate that local chemistry also influences ${\gamma }_{{}_{ISF}}$ in the axial Ising model. We also propose a new formula ${\gamma }_{{}_{ISF}}=5E_{ABABC}-5E_{ABC}$, which is similarly efficient, simpler, and more accurate compared to the widely used ${\gamma }_{{}_{ISF}}=E_{AB}+2E_{ABAC}-3E_{ABC}$. We tested the new formula on pure Ni, Ni-Co alloys, and Cr-Mn-Fe-Co-Ni high entropy alloys and found satisfactory results.