求助PDF
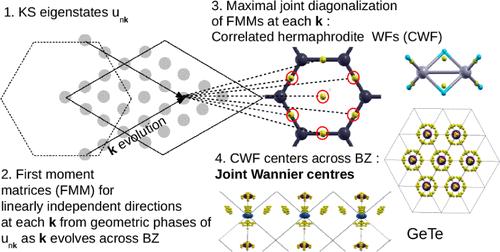
{"title":"从电子的几何相貌看物质中电荷中心的分布","authors":"Joyeta Saha, Sujith Nedungattil Subrahmanian, Joydeep Bhattacharjee","doi":"10.1021/acs.jpcc.4c05248","DOIUrl":null,"url":null,"abstract":"Based on the geometric phases of Bloch electrons, we propose a scheme for the unambiguous spatial partitioning of charge in matter from first-principles, derivable directly from the Kohn–Sham states. Generalizing the fact that geometric phases acquired by electrons, due to the evolution of their crystal momentum <i></i><span style=\"color: inherit;\"></span><span data-mathml='<math xmlns=\"http://www.w3.org/1998/Math/MathML\" display=\"inline\"><mover><mi>k</mi><mo>&#x2192;</mo></mover></math>' role=\"presentation\" style=\"position: relative;\" tabindex=\"0\"><nobr aria-hidden=\"true\"><span style=\"width: 1.026em; display: inline-block;\"><span style=\"display: inline-block; position: relative; width: 0.912em; height: 0px; font-size: 110%;\"><span style=\"position: absolute; clip: rect(0.798em, 1000.86em, 2.332em, -999.997em); top: -2.156em; left: 0em;\"><span><span><span style=\"display: inline-block; position: relative; width: 0.912em; height: 0px;\"><span style=\"position: absolute; clip: rect(3.128em, 1000.51em, 4.151em, -999.997em); top: -3.974em; left: 0.173em;\"><span style=\"font-family: STIXMathJax_Normal-italic;\">𝑘</span><span style=\"display: inline-block; width: 0px; height: 3.98em;\"></span></span><span style=\"position: absolute; clip: rect(3.355em, 1000.86em, 4.094em, -999.997em); top: -4.713em; left: 0em;\"><span style=\"\"><span style=\"font-family: STIXMathJax_Main;\">→</span></span><span style=\"display: inline-block; width: 0px; height: 3.98em;\"></span></span></span></span></span><span style=\"display: inline-block; width: 0px; height: 2.162em;\"></span></span></span><span style=\"display: inline-block; overflow: hidden; vertical-align: -0.059em; border-left: 0px solid; width: 0px; height: 1.441em;\"></span></span></nobr><span role=\"presentation\"><math display=\"inline\" xmlns=\"http://www.w3.org/1998/Math/MathML\"><mover><mi>k</mi><mo>→</mo></mover></math></span></span><script type=\"math/mml\"><math display=\"inline\"><mover><mi>k</mi><mo>→</mo></mover></math></script> in any arbitrary direction throughout the Brillouin zone (BZ), render the location of their spatial localization with net minimum spread along the direction in real space reciprocal to that of the evolution of <i></i><span style=\"color: inherit;\"></span><span data-mathml='<math xmlns=\"http://www.w3.org/1998/Math/MathML\" display=\"inline\"><mover><mi>k</mi><mo>&#x2192;</mo></mover></math>' role=\"presentation\" style=\"position: relative;\" tabindex=\"0\"><nobr aria-hidden=\"true\"><span style=\"width: 1.026em; display: inline-block;\"><span style=\"display: inline-block; position: relative; width: 0.912em; height: 0px; font-size: 110%;\"><span style=\"position: absolute; clip: rect(0.798em, 1000.86em, 2.332em, -999.997em); top: -2.156em; left: 0em;\"><span><span><span style=\"display: inline-block; position: relative; width: 0.912em; height: 0px;\"><span style=\"position: absolute; clip: rect(3.128em, 1000.51em, 4.151em, -999.997em); top: -3.974em; left: 0.173em;\"><span style=\"font-family: STIXMathJax_Normal-italic;\">𝑘</span><span style=\"display: inline-block; width: 0px; height: 3.98em;\"></span></span><span style=\"position: absolute; clip: rect(3.355em, 1000.86em, 4.094em, -999.997em); top: -4.713em; left: 0em;\"><span style=\"\"><span style=\"font-family: STIXMathJax_Main;\">→</span></span><span style=\"display: inline-block; width: 0px; height: 3.98em;\"></span></span></span></span></span><span style=\"display: inline-block; width: 0px; height: 2.162em;\"></span></span></span><span style=\"display: inline-block; overflow: hidden; vertical-align: -0.059em; border-left: 0px solid; width: 0px; height: 1.441em;\"></span></span></nobr><span role=\"presentation\"><math display=\"inline\" xmlns=\"http://www.w3.org/1998/Math/MathML\"><mover><mi>k</mi><mo>→</mo></mover></math></span></span><script type=\"math/mml\"><math display=\"inline\"><mover><mi>k</mi><mo>→</mo></mover></math></script>, we find that the total charge can be meaningfully distributed into centers of a class of correlated hermaphrodite Wannier functions simultaneously contributed by electrons with their crystal momenta evolving linearly independently through each unique <i></i><span style=\"color: inherit;\"></span><span data-mathml='<math xmlns=\"http://www.w3.org/1998/Math/MathML\" display=\"inline\"><mover><mi>k</mi><mo>&#x2192;</mo></mover></math>' role=\"presentation\" style=\"position: relative;\" tabindex=\"0\"><nobr aria-hidden=\"true\"><span style=\"width: 1.026em; display: inline-block;\"><span style=\"display: inline-block; position: relative; width: 0.912em; height: 0px; font-size: 110%;\"><span style=\"position: absolute; clip: rect(0.798em, 1000.86em, 2.332em, -999.997em); top: -2.156em; left: 0em;\"><span><span><span style=\"display: inline-block; position: relative; width: 0.912em; height: 0px;\"><span style=\"position: absolute; clip: rect(3.128em, 1000.51em, 4.151em, -999.997em); top: -3.974em; left: 0.173em;\"><span style=\"font-family: STIXMathJax_Normal-italic;\">𝑘</span><span style=\"display: inline-block; width: 0px; height: 3.98em;\"></span></span><span style=\"position: absolute; clip: rect(3.355em, 1000.86em, 4.094em, -999.997em); top: -4.713em; left: 0em;\"><span style=\"\"><span style=\"font-family: STIXMathJax_Main;\">→</span></span><span style=\"display: inline-block; width: 0px; height: 3.98em;\"></span></span></span></span></span><span style=\"display: inline-block; width: 0px; height: 2.162em;\"></span></span></span><span style=\"display: inline-block; overflow: hidden; vertical-align: -0.059em; border-left: 0px solid; width: 0px; height: 1.441em;\"></span></span></nobr><span role=\"presentation\"><math display=\"inline\" xmlns=\"http://www.w3.org/1998/Math/MathML\"><mover><mi>k</mi><mo>→</mo></mover></math></span></span><script type=\"math/mml\"><math display=\"inline\"><mover><mi>k</mi><mo>→</mo></mover></math></script> across the BZ. The resultant map of charge centers readily renders not only the qualitative nature of interatomic as well as intra-atomic hybridization of electrons but also unbiased quantitative estimates of electrons that can be associated with atoms or shared between them, as demonstrated in a selected variety of isolated and periodic systems with varying degrees of sharing of valence electrons among atoms, including variants of multicentered bonds.","PeriodicalId":61,"journal":{"name":"The Journal of Physical Chemistry C","volume":"26 1","pages":""},"PeriodicalIF":3.3000,"publicationDate":"2024-10-11","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"Distribution of Charge Centers in Matter from Geometric Phases of Electrons\",\"authors\":\"Joyeta Saha, Sujith Nedungattil Subrahmanian, Joydeep Bhattacharjee\",\"doi\":\"10.1021/acs.jpcc.4c05248\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"Based on the geometric phases of Bloch electrons, we propose a scheme for the unambiguous spatial partitioning of charge in matter from first-principles, derivable directly from the Kohn–Sham states. Generalizing the fact that geometric phases acquired by electrons, due to the evolution of their crystal momentum <i></i><span style=\\\"color: inherit;\\\"></span><span data-mathml='<math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\" display=\\\"inline\\\"><mover><mi>k</mi><mo>&#x2192;</mo></mover></math>' role=\\\"presentation\\\" style=\\\"position: relative;\\\" tabindex=\\\"0\\\"><nobr aria-hidden=\\\"true\\\"><span style=\\\"width: 1.026em; display: inline-block;\\\"><span style=\\\"display: inline-block; position: relative; width: 0.912em; height: 0px; font-size: 110%;\\\"><span style=\\\"position: absolute; clip: rect(0.798em, 1000.86em, 2.332em, -999.997em); top: -2.156em; left: 0em;\\\"><span><span><span style=\\\"display: inline-block; position: relative; width: 0.912em; height: 0px;\\\"><span style=\\\"position: absolute; clip: rect(3.128em, 1000.51em, 4.151em, -999.997em); top: -3.974em; left: 0.173em;\\\"><span style=\\\"font-family: STIXMathJax_Normal-italic;\\\">𝑘</span><span style=\\\"display: inline-block; width: 0px; height: 3.98em;\\\"></span></span><span style=\\\"position: absolute; clip: rect(3.355em, 1000.86em, 4.094em, -999.997em); top: -4.713em; left: 0em;\\\"><span style=\\\"\\\"><span style=\\\"font-family: STIXMathJax_Main;\\\">→</span></span><span style=\\\"display: inline-block; width: 0px; height: 3.98em;\\\"></span></span></span></span></span><span style=\\\"display: inline-block; width: 0px; height: 2.162em;\\\"></span></span></span><span style=\\\"display: inline-block; overflow: hidden; vertical-align: -0.059em; border-left: 0px solid; width: 0px; height: 1.441em;\\\"></span></span></nobr><span role=\\\"presentation\\\"><math display=\\\"inline\\\" xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><mover><mi>k</mi><mo>→</mo></mover></math></span></span><script type=\\\"math/mml\\\"><math display=\\\"inline\\\"><mover><mi>k</mi><mo>→</mo></mover></math></script> in any arbitrary direction throughout the Brillouin zone (BZ), render the location of their spatial localization with net minimum spread along the direction in real space reciprocal to that of the evolution of <i></i><span style=\\\"color: inherit;\\\"></span><span data-mathml='<math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\" display=\\\"inline\\\"><mover><mi>k</mi><mo>&#x2192;</mo></mover></math>' role=\\\"presentation\\\" style=\\\"position: relative;\\\" tabindex=\\\"0\\\"><nobr aria-hidden=\\\"true\\\"><span style=\\\"width: 1.026em; display: inline-block;\\\"><span style=\\\"display: inline-block; position: relative; width: 0.912em; height: 0px; font-size: 110%;\\\"><span style=\\\"position: absolute; clip: rect(0.798em, 1000.86em, 2.332em, -999.997em); top: -2.156em; left: 0em;\\\"><span><span><span style=\\\"display: inline-block; position: relative; width: 0.912em; height: 0px;\\\"><span style=\\\"position: absolute; clip: rect(3.128em, 1000.51em, 4.151em, -999.997em); top: -3.974em; left: 0.173em;\\\"><span style=\\\"font-family: STIXMathJax_Normal-italic;\\\">𝑘</span><span style=\\\"display: inline-block; width: 0px; height: 3.98em;\\\"></span></span><span style=\\\"position: absolute; clip: rect(3.355em, 1000.86em, 4.094em, -999.997em); top: -4.713em; left: 0em;\\\"><span style=\\\"\\\"><span style=\\\"font-family: STIXMathJax_Main;\\\">→</span></span><span style=\\\"display: inline-block; width: 0px; height: 3.98em;\\\"></span></span></span></span></span><span style=\\\"display: inline-block; width: 0px; height: 2.162em;\\\"></span></span></span><span style=\\\"display: inline-block; overflow: hidden; vertical-align: -0.059em; border-left: 0px solid; width: 0px; height: 1.441em;\\\"></span></span></nobr><span role=\\\"presentation\\\"><math display=\\\"inline\\\" xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><mover><mi>k</mi><mo>→</mo></mover></math></span></span><script type=\\\"math/mml\\\"><math display=\\\"inline\\\"><mover><mi>k</mi><mo>→</mo></mover></math></script>, we find that the total charge can be meaningfully distributed into centers of a class of correlated hermaphrodite Wannier functions simultaneously contributed by electrons with their crystal momenta evolving linearly independently through each unique <i></i><span style=\\\"color: inherit;\\\"></span><span data-mathml='<math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\" display=\\\"inline\\\"><mover><mi>k</mi><mo>&#x2192;</mo></mover></math>' role=\\\"presentation\\\" style=\\\"position: relative;\\\" tabindex=\\\"0\\\"><nobr aria-hidden=\\\"true\\\"><span style=\\\"width: 1.026em; display: inline-block;\\\"><span style=\\\"display: inline-block; position: relative; width: 0.912em; height: 0px; font-size: 110%;\\\"><span style=\\\"position: absolute; clip: rect(0.798em, 1000.86em, 2.332em, -999.997em); top: -2.156em; left: 0em;\\\"><span><span><span style=\\\"display: inline-block; position: relative; width: 0.912em; height: 0px;\\\"><span style=\\\"position: absolute; clip: rect(3.128em, 1000.51em, 4.151em, -999.997em); top: -3.974em; left: 0.173em;\\\"><span style=\\\"font-family: STIXMathJax_Normal-italic;\\\">𝑘</span><span style=\\\"display: inline-block; width: 0px; height: 3.98em;\\\"></span></span><span style=\\\"position: absolute; clip: rect(3.355em, 1000.86em, 4.094em, -999.997em); top: -4.713em; left: 0em;\\\"><span style=\\\"\\\"><span style=\\\"font-family: STIXMathJax_Main;\\\">→</span></span><span style=\\\"display: inline-block; width: 0px; height: 3.98em;\\\"></span></span></span></span></span><span style=\\\"display: inline-block; width: 0px; height: 2.162em;\\\"></span></span></span><span style=\\\"display: inline-block; overflow: hidden; vertical-align: -0.059em; border-left: 0px solid; width: 0px; height: 1.441em;\\\"></span></span></nobr><span role=\\\"presentation\\\"><math display=\\\"inline\\\" xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><mover><mi>k</mi><mo>→</mo></mover></math></span></span><script type=\\\"math/mml\\\"><math display=\\\"inline\\\"><mover><mi>k</mi><mo>→</mo></mover></math></script> across the BZ. The resultant map of charge centers readily renders not only the qualitative nature of interatomic as well as intra-atomic hybridization of electrons but also unbiased quantitative estimates of electrons that can be associated with atoms or shared between them, as demonstrated in a selected variety of isolated and periodic systems with varying degrees of sharing of valence electrons among atoms, including variants of multicentered bonds.\",\"PeriodicalId\":61,\"journal\":{\"name\":\"The Journal of Physical Chemistry C\",\"volume\":\"26 1\",\"pages\":\"\"},\"PeriodicalIF\":3.3000,\"publicationDate\":\"2024-10-11\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"The Journal of Physical Chemistry C\",\"FirstCategoryId\":\"1\",\"ListUrlMain\":\"https://doi.org/10.1021/acs.jpcc.4c05248\",\"RegionNum\":3,\"RegionCategory\":\"化学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q2\",\"JCRName\":\"CHEMISTRY, PHYSICAL\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"The Journal of Physical Chemistry C","FirstCategoryId":"1","ListUrlMain":"https://doi.org/10.1021/acs.jpcc.4c05248","RegionNum":3,"RegionCategory":"化学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q2","JCRName":"CHEMISTRY, PHYSICAL","Score":null,"Total":0}
引用次数: 0
引用
批量引用

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


