在 $$\mathbb {R}^n\rtimes \textrm{SL}_2(\mathbb {R})$$ 中的网格

Pub Date : 2024-08-07 DOI:10.1007/s00031-024-09874-z

M. M. Radhika, Sandip Singh

引用次数: 0

摘要

我们确定了在$\textrm{V}\rtimes \textrm{SL}_2(\mathbb {R})$形式的群中cocompact网格的存在性，其中$\textrm{V}$是$\textrm{SL}_2(\mathbb {R})$的有限维实数表示。事实证明，当表示是不可还原的时候，答案取决于 $\dim (\textrm{V})$ 的奇偶性。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

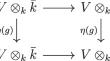
$Lattices in $$\mathbb {R}^n\rtimes \textrm{SL}_2(\mathbb {R})$$$

查看原文

Lattices in $$\mathbb {R}^n\rtimes \textrm{SL}_2(\mathbb {R})$$

We determine the existence of cocompact lattices in groups of the form $\textrm{V}\rtimes \textrm{SL}_2(\mathbb {R})$, where $\textrm{V}$ is a finite dimensional real representation of $\textrm{SL}_2(\mathbb {R})$. It turns out that the answer depends on the parity of $\dim (\textrm{V})$ when the representation is irreducible.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助