带有 Probit 类型目标的连续等价包
IF 1.5
3区 数学
Q2 MATHEMATICS, APPLIED
Journal of Optimization Theory and Applications
Pub Date : 2024-08-12
DOI:10.1007/s10957-024-02503-5
引用次数: 0
摘要
我们研究的是连续、相等、具有均匀可分、非凸目标函数的knapsack问题,这些目标函数是连续的、关于一点的非对称的,并且有凹和凸区域。例如,该模型捕捉了一个简单的分配问题,其目标是优化预期值,目标函数是同分布正态分布的累积分布函数之和(即逆概率函数之和)。我们证明了该模型在一般假设下的结构性结果,并提供了两种高效优化算法:(1) 在线性时间内运行;(2) 在对目标函数进行预处理的情况下,以恒定的运算次数运行。本文章由计算机程序翻译,如有差异,请以英文原文为准。
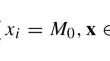
Continuous Equality Knapsack with Probit-Style Objectives
We study continuous, equality knapsack problems with uniform separable, non-convex objective functions that are continuous, antisymmetric about a point, and have concave and convex regions. For example, this model captures a simple allocation problem with the goal of optimizing an expected value where the objective is a sum of cumulative distribution functions of identically distributed normal distributions (i.e., a sum of inverse probit functions). We prove structural results of this model under general assumptions and provide two algorithms for efficient optimization: (1) running in linear time and (2) running in a constant number of operations given preprocessing of the objective function.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
3.30
自引率
5.30%
发文量
149
审稿时长
9.9 months
期刊介绍:
The Journal of Optimization Theory and Applications is devoted to the publication of carefully selected regular papers, invited papers, survey papers, technical notes, book notices, and forums that cover mathematical optimization techniques and their applications to science and engineering. Typical theoretical areas include linear, nonlinear, mathematical, and dynamic programming. Among the areas of application covered are mathematical economics, mathematical physics and biology, and aerospace, chemical, civil, electrical, and mechanical engineering.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


