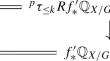
The $$P=W$$ identity for isolated cluster varieties: full rank case

We initiate a systematic construction of real analytic Lagrangian fibrations from integer matrices. We prove that when the matrix is of full column rank, the perverse filtration associated with the Lagrangian fibration matches the mixed Hodge-theoretic weight filtration of the isolated cluster variety associated with the matrix.