双相不平衡增长特征值问题的连续谱

IF 1.1 3区数学 Q1 MATHEMATICS

Mediterranean Journal of Mathematics Pub Date : 2024-05-17 DOI:10.1007/s00009-024-02664-7

Laura Gambera, Umberto Guarnotta, Nikolaos S. Papageorgiou

引用次数: 0

摘要

我们考虑了一个不平衡增长的双相微分算子的特征值问题。通过使用 Nehari 方法，我们证明了该问题具有由加权 p 拉普拉奇的最小特征值决定的连续谱。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

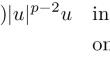
Continuous Spectrum for a Double-Phase Unbalanced Growth Eigenvalue Problem

查看原文本刊更多论文

Continuous Spectrum for a Double-Phase Unbalanced Growth Eigenvalue Problem

We consider an eigenvalue problem for a double-phase differential operator with unbalanced growth. Using the Nehari method, we show that the problem has a continuous spectrum determined by the minimal eigenvalue of the weighted p-Laplacian.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Mediterranean Journal of Mathematics 数学-数学

CiteScore

1.80

自引率

0.00%

发文量

261

审稿时长

6-12 weeks

期刊介绍： The Mediterranean Journal of Mathematics (MedJM) is a publication issued by the Department of Mathematics of the University of Bari. The new journal replaces the Conferenze del Seminario di Matematica dell’Università di Bari which has been in publication from 1954 until 2003. The Mediterranean Journal of Mathematics aims to publish original and high-quality peer-reviewed papers containing significant results across all fields of mathematics. The submitted papers should be of medium length (not to exceed 20 printed pages), well-written and appealing to a broad mathematical audience. In particular, the Mediterranean Journal of Mathematics intends to offer mathematicians from the Mediterranean countries a particular opportunity to circulate the results of their researches in a common journal. Through such a new cultural and scientific stimulus the journal aims to contribute to further integration amongst Mediterranean universities, though it is open to contribution from mathematicians across the world.