具有非零边界条件的聚焦耦合修正科特韦格-德-弗里斯方程:黎曼-希尔伯特问题与孤子分类
IF 1
4区 物理与天体物理
Q3 PHYSICS, MATHEMATICAL
引用次数: 0
摘要
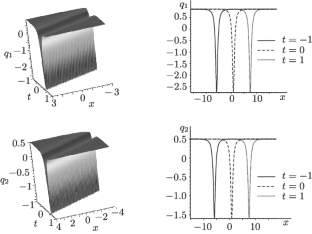
摘要 采用黎曼-希尔伯特方法研究了具有非零边界条件的聚焦耦合修正 Korteweg-de Vries 方程。通过三个对称性推导出紧凑的精确解。这些解包括六种不同类型的孤子解和呼吸器解,如暗-暗、亮-亮、磕-暗-暗、磕-亮-亮孤子,以及呼吸器-呼吸器解。本文章由计算机程序翻译,如有差异,请以英文原文为准。
The focusing coupled modified Korteweg–de Vries equation with nonzero boundary conditions: the Riemann–Hilbert problem and soliton classification
The focusing coupled modified Korteweg–de Vries equation with nonzero boundary conditions is investigated by the Riemann–Hilbert approach. Three symmetries are formulated to derive compact exact solutions. The solutions include six different types of soliton solutions and breathers, such as dark–dark, bright–bright, kink–dark–dark, kink–bright–bright solitons, and a breather–breather solution.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Theoretical and Mathematical Physics
物理-物理:数学物理
CiteScore
1.60
自引率
20.00%
发文量
103
审稿时长
4-8 weeks
期刊介绍:
Theoretical and Mathematical Physics covers quantum field theory and theory of elementary particles, fundamental problems of nuclear physics, many-body problems and statistical physics, nonrelativistic quantum mechanics, and basic problems of gravitation theory. Articles report on current developments in theoretical physics as well as related mathematical problems.
Theoretical and Mathematical Physics is published in collaboration with the Steklov Mathematical Institute of the Russian Academy of Sciences.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


