科恩-麦考莱加权定向弦图和单纯图
IF 0.5
4区 数学
Q3 MATHEMATICS
引用次数: 0
摘要
Herzog、Hibi 和 Zheng 对弦图的 Cohen-Macaulay 边理想进行了分类。在本文中,我们对(顶点)加权定向弦图和单纯图的 Cohen-Macaulay 边理想进行了分类,这是一类更普遍的单项式理想。特别是,我们证明了这些理想的 Cohen-Macaulay 性质等同于非混合性质,因此与底层域无关。本文章由计算机程序翻译,如有差异,请以英文原文为准。
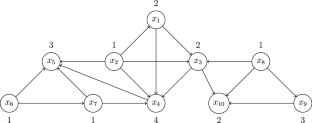

Cohen-Macaulay weighted oriented chordal and simplicial graphs
Herzog, Hibi, and Zheng classified the Cohen-Macaulay edge ideals of chordal graphs. In this paper, we classify Cohen-Macaulay edge ideals of (vertex) weighted oriented chordal and simplicial graphs, a more general class of monomial ideals. In particular, we show that the Cohen-Macaulay property of these ideals is equivalent to the unmixed one and hence, independent of the underlying field.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Archiv der Mathematik
数学-数学
CiteScore
1.10
自引率
0.00%
发文量
117
审稿时长
4-8 weeks
期刊介绍:
Archiv der Mathematik (AdM) publishes short high quality research papers in every area of mathematics which are not overly technical in nature and addressed to a broad readership.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


