论生物进化模型中出现的科尔莫戈罗夫-费勒方程的解法

IF 0.2 Q4 MATHEMATICS

Moscow University Mathematics Bulletin Pub Date : 2024-03-24 DOI:10.3103/s0027132223060062

O. S. Rozanova

{"title":"论生物进化模型中出现的科尔莫戈罗夫-费勒方程的解法","authors":"O. S. Rozanova","doi":"10.3103/s0027132223060062","DOIUrl":null,"url":null,"abstract":"<h3 data-test=\"abstract-sub-heading\">Abstract</h3><p>The paper considers Kolmogorov–Feller equation for the probability density of a Markov process on a half-axis, which arises in important problems of biology. This process consists of random jumps distributed according to the Laplace law and a deterministic return to zero. It is shown that the Green function for such an equation can be found both in the form of a series and in explicit form for some ratios of the parameters. This allows finding explicit solutions to the Kolmogorov–Feller equation for many initial data.</p>","PeriodicalId":42963,"journal":{"name":"Moscow University Mathematics Bulletin","volume":null,"pages":null},"PeriodicalIF":0.2000,"publicationDate":"2024-03-24","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"On the Solution to the Kolmogorov-Feller Equation Arising in a Biological Evolution Model\",\"authors\":\"O. S. Rozanova\",\"doi\":\"10.3103/s0027132223060062\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<h3 data-test=\\\"abstract-sub-heading\\\">Abstract</h3><p>The paper considers Kolmogorov–Feller equation for the probability density of a Markov process on a half-axis, which arises in important problems of biology. This process consists of random jumps distributed according to the Laplace law and a deterministic return to zero. It is shown that the Green function for such an equation can be found both in the form of a series and in explicit form for some ratios of the parameters. This allows finding explicit solutions to the Kolmogorov–Feller equation for many initial data.</p>\",\"PeriodicalId\":42963,\"journal\":{\"name\":\"Moscow University Mathematics Bulletin\",\"volume\":null,\"pages\":null},\"PeriodicalIF\":0.2000,\"publicationDate\":\"2024-03-24\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Moscow University Mathematics Bulletin\",\"FirstCategoryId\":\"1085\",\"ListUrlMain\":\"https://doi.org/10.3103/s0027132223060062\",\"RegionNum\":0,\"RegionCategory\":null,\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q4\",\"JCRName\":\"MATHEMATICS\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Moscow University Mathematics Bulletin","FirstCategoryId":"1085","ListUrlMain":"https://doi.org/10.3103/s0027132223060062","RegionNum":0,"RegionCategory":null,"ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q4","JCRName":"MATHEMATICS","Score":null,"Total":0}

引用次数: 0

摘要

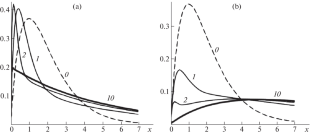
摘要本文研究了半轴上马尔可夫过程概率密度的科尔莫哥罗德-费勒方程，该过程出现在重要的生物学问题中。该过程由根据拉普拉斯定律分布的随机跳跃和确定性归零组成。研究表明，这种方程的格林函数既可以数列形式求得，也可以某些参数比的显式形式求得。这样就可以为许多初始数据找到科尔莫哥罗德-费勒方程的显式解。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

On the Solution to the Kolmogorov-Feller Equation Arising in a Biological Evolution Model

查看原文本刊更多论文

On the Solution to the Kolmogorov-Feller Equation Arising in a Biological Evolution Model

Abstract

The paper considers Kolmogorov–Feller equation for the probability density of a Markov process on a half-axis, which arises in important problems of biology. This process consists of random jumps distributed according to the Laplace law and a deterministic return to zero. It is shown that the Green function for such an equation can be found both in the form of a series and in explicit form for some ratios of the parameters. This allows finding explicit solutions to the Kolmogorov–Feller equation for many initial data.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Moscow University Mathematics Bulletin MATHEMATICS-

CiteScore

0.60

自引率

25.00%

发文量

期刊介绍： Moscow University Mathematics Bulletin is the journal of scientific publications reflecting the most important areas of mathematical studies at Lomonosov Moscow State University. The journal covers research in theory of functions, functional analysis, algebra, geometry, topology, ordinary and partial differential equations, probability theory, stochastic processes, mathematical statistics, optimal control, number theory, mathematical logic, theory of algorithms, discrete mathematics and computational mathematics.