通过混合整数编程研究紧凑集上极化问题的界限
IF 0.6
3区 数学
Q4 COMPUTER SCIENCE, THEORY & METHODS
引用次数: 0
摘要
寻找能产生最大极化(切比雪夫常数)的点配置在几何优化领域越来越受到关注。在本文中,我们将研究紧凑集上无约束最大极化问题。我们特别讨论了局部最优的必要条件,例如局部最优配置总是包含在各自最暗点的凸壳中。在此基础上,我们提出了两个混合整数线性程序序列,以计算最大极化的下限和上限,其中下限是构造性的。此外,我们还证明了这些序列对最大极化的收敛性。本文章由计算机程序翻译,如有差异,请以英文原文为准。
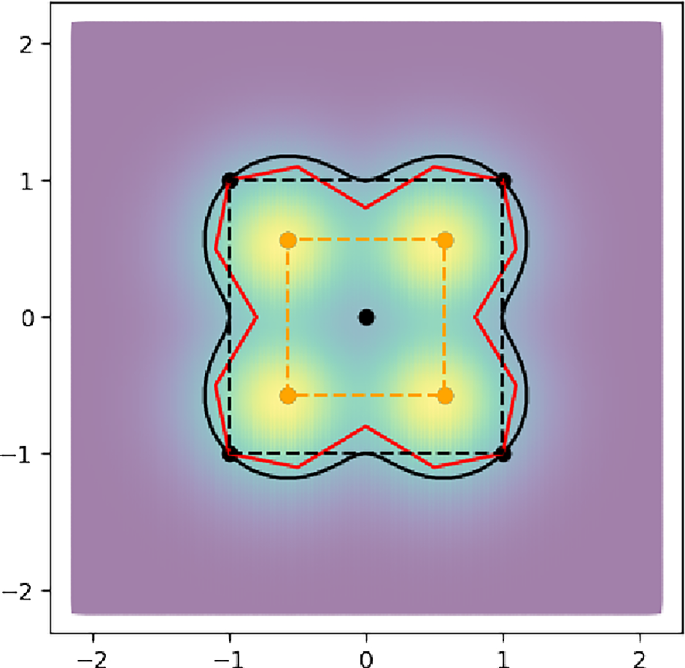
Bounds on Polarization Problems on Compact Sets via Mixed Integer Programming
Finding point configurations, that yield the maximum polarization (Chebyshev constant) is gaining interest in the field of geometric optimization. In the present article, we study the problem of unconstrained maximum polarization on compact sets. In particular, we discuss necessary conditions for local optimality, such as that a locally optimal configuration is always contained in the convex hull of the respective darkest points. Building on this, we propose two sequences of mixed-integer linear programs in order to compute lower and upper bounds on the maximal polarization, where the lower bound is constructive. Moreover, we prove the convergence of these sequences towards the maximal polarization.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Discrete & Computational Geometry
数学-计算机:理论方法
CiteScore
1.80
自引率
12.50%
发文量
99
审稿时长
6-12 weeks
期刊介绍:
Discrete & Computational Geometry (DCG) is an international journal of mathematics and computer science, covering a broad range of topics in which geometry plays a fundamental role. It publishes papers on such topics as configurations and arrangements, spatial subdivision, packing, covering, and tiling, geometric complexity, polytopes, point location, geometric probability, geometric range searching, combinatorial and computational topology, probabilistic techniques in computational geometry, geometric graphs, geometry of numbers, and motion planning.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


