标志域中碱基循环正常束的放大率
IF 0.4
3区 数学
Q4 MATHEMATICS
引用次数: 0
摘要
旗域是作用于旗流形的复半简单李群的非紧凑实形式的开放轨道。每个旗域都可以关联一个称为基周期的紧凑复流形。旗域中基底周期法线束的振幅度量了基底周期附近的凹性。在本文中,我们计算了各种情况下旗域中基底周期法线束的振幅,包括 G 为经典时全旗流形 G/B 中的旗域,以及具有固定霍奇数的参数化极化霍奇结构的周期域。本文章由计算机程序翻译,如有差异,请以英文原文为准。
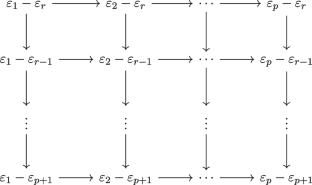
Ampleness of Normal Bundles of Base Cycles in Flag Domains
Flag domains are open orbits of noncompact real forms of complex semisimple Lie groups acting on flag manifolds. To each flag domain one can associate a compact complex manifold called the base cycle. The ampleness of the normal bundle of the base cycle in a flag domain measures the concavity near the base cycle. In this paper we compute the ampleness of normal bundles of base cycles in flag domains in various cases, including flag domains in the full flag manifolds G/B when G is classical, and period domains parameterizing polarized Hodge structures with fixed Hodge numbers.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Transformation Groups
数学-数学
CiteScore
1.60
自引率
0.00%
发文量
100
审稿时长
9 months
期刊介绍:
Transformation Groups will only accept research articles containing new results, complete Proofs, and an abstract. Topics include: Lie groups and Lie algebras; Lie transformation groups and holomorphic transformation groups; Algebraic groups; Invariant theory; Geometry and topology of homogeneous spaces; Discrete subgroups of Lie groups; Quantum groups and enveloping algebras; Group aspects of conformal field theory; Kac-Moody groups and algebras; Lie supergroups and superalgebras.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


