图与1-和

IF 0.6 4区数学 Q3 MATHEMATICS

Graphs and Combinatorics Pub Date : 2023-12-04 DOI:10.1007/s00373-023-02730-z

Jagdeep Singh

{"title":"图与1-和","authors":"Jagdeep Singh","doi":"10.1007/s00373-023-02730-z","DOIUrl":null,"url":null,"abstract":"A graph that can be generated from \\(K_1\\) using joins and 0-sums is called a cograph. We define a sesquicograph to be a graph that can be generated from \\(K_1\\) using joins, 0-sums, and 1-sums. We show that, like cographs, sesquicographs are closed under induced minors. Cographs are precisely the graphs that do not have the 4-vertex path as an induced subgraph. We obtain an analogue of this result for sesquicographs, that is, we find those non-sesquicographs for which every proper induced subgraph is a sesquicograph.","PeriodicalId":12811,"journal":{"name":"Graphs and Combinatorics","volume":null,"pages":null},"PeriodicalIF":0.6000,"publicationDate":"2023-12-04","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"Cographs and 1-Sums\",\"authors\":\"Jagdeep Singh\",\"doi\":\"10.1007/s00373-023-02730-z\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"A graph that can be generated from \\\\(K_1\\\\) using joins and 0-sums is called a cograph. We define a sesquicograph to be a graph that can be generated from \\\\(K_1\\\\) using joins, 0-sums, and 1-sums. We show that, like cographs, sesquicographs are closed under induced minors. Cographs are precisely the graphs that do not have the 4-vertex path as an induced subgraph. We obtain an analogue of this result for sesquicographs, that is, we find those non-sesquicographs for which every proper induced subgraph is a sesquicograph.\",\"PeriodicalId\":12811,\"journal\":{\"name\":\"Graphs and Combinatorics\",\"volume\":null,\"pages\":null},\"PeriodicalIF\":0.6000,\"publicationDate\":\"2023-12-04\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Graphs and Combinatorics\",\"FirstCategoryId\":\"100\",\"ListUrlMain\":\"https://doi.org/10.1007/s00373-023-02730-z\",\"RegionNum\":4,\"RegionCategory\":\"数学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q3\",\"JCRName\":\"MATHEMATICS\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Graphs and Combinatorics","FirstCategoryId":"100","ListUrlMain":"https://doi.org/10.1007/s00373-023-02730-z","RegionNum":4,"RegionCategory":"数学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q3","JCRName":"MATHEMATICS","Score":null,"Total":0}

引用次数: 0

摘要

可以使用连接和0和从\(K_1\)生成的图称为cograph。我们将sesquicgraph定义为可以使用连接、0和和和和从\(K_1\)生成的图。我们证明，像图形一样，sesquicographs在诱导未成年下是闭合的。图就是没有4顶点路径作为诱导子图的图。我们得到了这个结果在倍形图上的一个类似的结果，即我们找到了那些非倍形图，它们的每个固有诱导子图都是倍形图。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

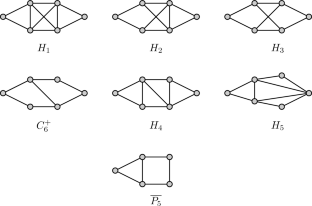
Cographs and 1-Sums

查看原文本刊更多论文

Cographs and 1-Sums

A graph that can be generated from \(K_1\) using joins and 0-sums is called a cograph. We define a sesquicograph to be a graph that can be generated from \(K_1\) using joins, 0-sums, and 1-sums. We show that, like cographs, sesquicographs are closed under induced minors. Cographs are precisely the graphs that do not have the 4-vertex path as an induced subgraph. We obtain an analogue of this result for sesquicographs, that is, we find those non-sesquicographs for which every proper induced subgraph is a sesquicograph.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Graphs and Combinatorics 数学-数学

CiteScore

1.00

自引率

14.30%

发文量

160

审稿时长

6 months

期刊介绍： Graphs and Combinatorics is an international journal devoted to research concerning all aspects of combinatorial mathematics. In addition to original research papers, the journal also features survey articles from authors invited by the editorial board.