无网格加权粒子法在Vlasov-Poisson方程中的应用
IF 2.2
2区 数学
Q1 MATHEMATICS, APPLIED
引用次数: 0
摘要
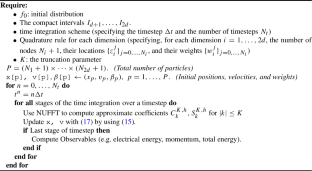
我们研究了一种基于跟踪Vlasov-Poisson系统特征演变的无网格粒子方法,并证明了它在足够光滑的初始数据下是收敛的。这种方法是建立在一个充分研究的构建模块的组合-主要是时间积分和积分正交-并允许获得任意高阶。通过使用非均匀快速傅里叶变换,总的计算复杂度是$$ {\mathcal {O}}(P \log P + K^d \log K^d) $$,其中$$ P $$是粒子的总数我们只保留傅里叶模式$$ k \in ({\mathbb {Z}}^d)^* $$这样$$ k_1^2 + \dots + k_d^2 \le K^2 $$。给出了一维情况下Vlasov-Poisson系统的一些数值结果。本文章由计算机程序翻译,如有差异,请以英文原文为准。
Grid-free weighted particle method applied to the Vlasov–Poisson equation
We study a grid-free particle method based on following the evolution of the characteristics of the Vlasov–Poisson system, and we show that it converges for smooth enough initial data. This method is built as a combination of well-studied building blocks—mainly time integration and integral quadratures—and allows to obtain arbitrarily high orders. By making use of the Non-Uniform Fast Fourier Transform, the overall computational complexity is $$ {\mathcal {O}}(P \log P + K^d \log K^d) $$ , where $$ P $$ is the total number of particles and where we only keep the Fourier modes $$ k \in ({\mathbb {Z}}^d)^* $$ such that $$ k_1^2 + \dots + k_d^2 \le K^2 $$ . Some numerical results are given for the Vlasov–Poisson system in the one-dimensional case.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Numerische Mathematik
数学-应用数学
CiteScore
4.10
自引率
4.80%
发文量
72
审稿时长
6-12 weeks
期刊介绍:
Numerische Mathematik publishes papers of the very highest quality presenting significantly new and important developments in all areas of Numerical Analysis. "Numerical Analysis" is here understood in its most general sense, as that part of Mathematics that covers:
1. The conception and mathematical analysis of efficient numerical schemes actually used on computers (the "core" of Numerical Analysis)
2. Optimization and Control Theory
3. Mathematical Modeling
4. The mathematical aspects of Scientific Computing
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


