维迪尔二象性成立的偏序集
IF 1.2
2区 数学
Q1 MATHEMATICS
引用次数: 3
摘要
讨论了两个已知的轴-协轴对偶定理:有限正则CW复合体的面偏集的Curry定理和紧Hausdorff空间的Lurie定理,即协变Verdier对偶。我们给出了它们的统一公式,并证明了它们的推广。前者的版本适用于球谱和更一般的有限偏序集,我们用Gorenstein*条件来描述它。我们对后者的解释是,在Gaitsgory意义上,适当分离的$$\infty $$∞-拓扑的稳定性是刚性的。作为应用,对于分层拓扑空间,我们澄清了这两个对偶等价之间的关系。本文章由计算机程序翻译,如有差异,请以英文原文为准。
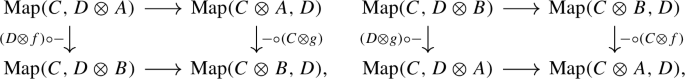
Posets for which Verdier duality holds
Abstract We discuss two known sheaf-cosheaf duality theorems: Curry’s for the face posets of finite regular CW complexes and Lurie’s for compact Hausdorff spaces, i.e., covariant Verdier duality. We provide a uniform formulation for them and prove their generalizations. Our version of the former works over the sphere spectrum and for more general finite posets, which we characterize in terms of the Gorenstein* condition. Our version of the latter says that the stabilization of a proper separated $$\infty $$ ∞ -topos is rigid in the sense of Gaitsgory. As an application, for stratified topological spaces, we clarify the relation between these two duality equivalences.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
2.30
自引率
7.10%
发文量
68
审稿时长
>12 weeks
期刊介绍:
Selecta Mathematica, New Series is a peer-reviewed journal addressed to a wide mathematical audience. It accepts well-written high quality papers in all areas of pure mathematics, and selected areas of applied mathematics. The journal especially encourages submission of papers which have the potential of opening new perspectives.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


