热导率对滑片附近卡森流体流动影响的评估:基于第六类切比雪夫多项式的数值模拟
IF 1.6
4区 物理与天体物理
Q2 MATHEMATICS, APPLIED
引用次数: 0
摘要
摘要本研究旨在阐明欧姆耗散和磁场对卡森流体在垂直拉伸表面上流动行为的影响。目标是用数值方法来解决这个问题。此外,流体的导热系数打算与温度成比例地变化。研究中考虑了热辐射、电场和粘性耗散的影响。用一组偏微分方程(PDEs)定量地反映了放置在薄板周围壁上的众多物理条件以及动量和热量传递过程。利用相似变换从常微分方程集生成常微分方程系统。通过这种转换,问题的数学模型变得更容易了。此外,本研究的主要目标是研究将卡普托分数阶导数考虑在内的拟议模型的数值处理。采用谱配点法求解变换后的ode系统。该方法利用第6类切比雪夫多项式逼近ode的解,有效地解决了该问题。通过若干观测来评价该方法的有效性,并研究了该方法的收敛性。不同参数对速度和温度分布的影响的可视化表示提供了对其影响的透彻理解。这些图形表示为了解系统在各种场景中的行为提供了深刻的见解。研究结果表明,混合对流参数和局部电参数对速度场都有促进作用。此外,温度场受到滑移速度、导热系数和埃克特数的积极影响。这些发现表明,改变这些变量将对系统的流体流动和传热性能产生影响。本文章由计算机程序翻译,如有差异,请以英文原文为准。
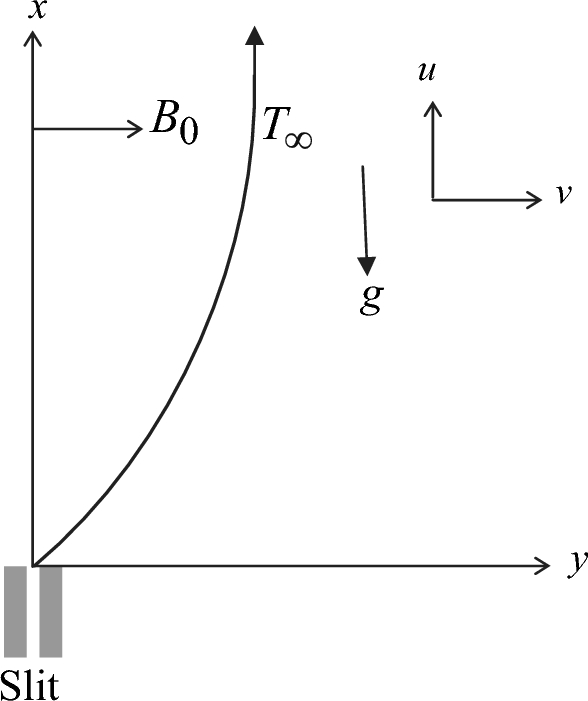
Evaluating the Impacts of Thermal Conductivity on Casson Fluid Flow Near a Slippery Sheet: Numerical Simulation Using Sixth-Kind Chebyshev Polynomials
Abstract This study aims to elucidates the effects of Ohmic dissipation and the magnetic field on the behavior of a Casson fluid flowing across a vertically stretched surface. The goal is to solve the problem by using numerical approaches. Furthermore, the fluid’s thermal conductivity is intended to vary proportionately with temperature. The effects of thermal radiation, electric fields, and viscous dissipation are taken into account in this study. A set of partial differential equations (PDEs) is used to quantitatively reflect the numerous physical conditions that are placed on the sheet’s surrounding wall as well as the processes of momentum and heat transport. A system of ordinary differential equations (ODEs) is created from the set of PDEs by using similarity transformations. The mathematical model of the problem is made easier by this conversion. Furthermore, this study’s main goal is to investigate the numerical treatment of the proposed model that takes Caputo fractional-order derivatives into account. The spectral collocation method is used to solve the system of ODEs that follow from the transformation. This approach efficiently solves the problem by approximating the solution of the ODEs using Chebyshev polynomials of the sixth kind. Several observations are made to evaluate the approach’s effectiveness, and the convergence of the method is studied. Visual representations of the effects of different parameters on the velocity and temperature profiles provide a thorough understanding of their effects. These graphical representations offer insightful views into how the system behaves in various scenarios. The results of this investigation suggest that the mixed convection parameter and the local electric parameter both boost the velocity field. Further, the temperature field is positively impacted by the slip velocity, thermal conductivity, and Eckert numbers. These findings imply that altering these variables will have an impact on the system’s fluid flow and heat transfer properties.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Journal of Nonlinear Mathematical Physics
PHYSICS, MATHEMATICAL-PHYSICS, MATHEMATICAL
CiteScore
1.60
自引率
0.00%
发文量
67
审稿时长
3 months
期刊介绍:
Journal of Nonlinear Mathematical Physics (JNMP) publishes research papers on fundamental mathematical and computational methods in mathematical physics in the form of Letters, Articles, and Review Articles.
Journal of Nonlinear Mathematical Physics is a mathematical journal devoted to the publication of research papers concerned with the description, solution, and applications of nonlinear problems in physics and mathematics.
The main subjects are:
-Nonlinear Equations of Mathematical Physics-
Quantum Algebras and Integrability-
Discrete Integrable Systems and Discrete Geometry-
Applications of Lie Group Theory and Lie Algebras-
Non-Commutative Geometry-
Super Geometry and Super Integrable System-
Integrability and Nonintegrability, Painleve Analysis-
Inverse Scattering Method-
Geometry of Soliton Equations and Applications of Twistor Theory-
Classical and Quantum Many Body Problems-
Deformation and Geometric Quantization-
Instanton, Monopoles and Gauge Theory-
Differential Geometry and Mathematical Physics
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


