具有奇异势的薄膜方程:接触线悖论的另一种解
IF 2.6
2区 数学
Q1 MATHEMATICS, APPLIED
引用次数: 0
摘要
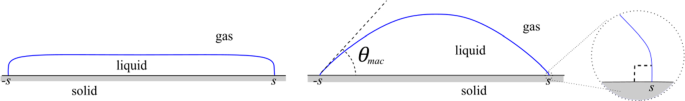
在润滑近似下,我们研究了奇异势作用下的扩散现象,其形式为$$P(h)\approx h^{1-m}$$ P (h)≈h1 - m: $$h\rightarrow 0^+$$ h→0 +,$$m>1$$ m &gt;1、模拟液气界面与衬底之间的斥力。我们假设接触线上的滑动为零。基于形式分析论证,我们报告了对于任意$$m>1$$ m &gt;1和速度的任何值(正负)都存在一个三参数,因此,一般的锋面族(即具有接触线的行波解)。一个推进的“线性-对数”锋面的双参数族也存在,在液体体中具有对数校正的线性行为。所有这些锋面的耗散率都是有限的,这表明奇异势可以作为接触线悖论的另一种解决办法。与稳态一致,对于所有$$m>1$$ m &gt,锋面的微观接触角等于$$\pi /2$$ π / 2;1和有限能量的所有$$m<3$$ m &lt;3 .我们还提出了一个选择标准的锋面,基于热力学一致的接触线条件,模拟在接触线上的摩擦。因此,就像滑移模型中的接触角条件一样,该准则为每个正速度选择一个唯一的(直到平移)线性对数前。数值证据表明,在一定的速度和摩擦系数下,其形状取决于扩散系数,部分润湿时锋面更陡,干燥完全润湿时前驱区更突出。本文章由计算机程序翻译,如有差异,请以英文原文为准。
Thin-Film Equations with Singular Potentials: An Alternative Solution to the Contact-Line Paradox
Abstract In the regime of lubrication approximation, we look at spreading phenomena under the action of singular potentials of the form $$P(h)\approx h^{1-m}$$ P ( h ) ≈ h 1 - m as $$h\rightarrow 0^+$$ h → 0 + with $$m>1$$ m > 1 , modeling repulsion between the liquid–gas interface and the substrate. We assume zero slippage at the contact line. Based on formal analysis arguments, we report that for any $$m>1$$ m > 1 and any value of the speed (both positive and negative) there exists a three-parameter, hence generic, family of fronts (i.e., traveling-wave solutions with a contact line). A two-parameter family of advancing “linear-log” fronts also exists, having a logarithmically corrected linear behavior in the liquid bulk. All these fronts have finite rate of dissipation, indicating that singular potentials stand as an alternative solution to the contact-line paradox. In agreement with steady states, fronts have microscopic contact angle equal to $$\pi /2$$ π / 2 for all $$m>1$$ m > 1 and finite energy for all $$m<3$$ m < 3 . We also propose a selection criterion for the fronts, based on thermodynamically consistent contact-line conditions modeling friction at the contact line. So as contact-angle conditions do in the case of slippage models, this criterion selects a unique (up to translation) linear-log front for each positive speed. Numerical evidence suggests that, fixed the speed and the frictional coefficient, its shape depends on the spreading coefficient, with steeper fronts in partial wetting and a more prominent precursor region in dry complete wetting.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
5.00
自引率
3.30%
发文量
87
审稿时长
4.5 months
期刊介绍:
The mission of the Journal of Nonlinear Science is to publish papers that augment the fundamental ways we describe, model, and predict nonlinear phenomena. Papers should make an original contribution to at least one technical area and should in addition illuminate issues beyond that area''s boundaries. Even excellent papers in a narrow field of interest are not appropriate for the journal. Papers can be oriented toward theory, experimentation, algorithms, numerical simulations, or applications as long as the work is creative and sound. Excessively theoretical work in which the application to natural phenomena is not apparent (at least through similar techniques) or in which the development of fundamental methodologies is not present is probably not appropriate. In turn, papers oriented toward experimentation, numerical simulations, or applications must not simply report results without an indication of what a theoretical explanation might be.
All papers should be submitted in English and must meet common standards of usage and grammar. In addition, because ours is a multidisciplinary subject, at minimum the introduction to the paper should be readable to a broad range of scientists and not only to specialists in the subject area. The scientific importance of the paper and its conclusions should be made clear in the introduction-this means that not only should the problem you study be presented, but its historical background, its relevance to science and technology, the specific phenomena it can be used to describe or investigate, and the outstanding open issues related to it should be explained. Failure to achieve this could disqualify the paper.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


