多维:简单游戏的维度扩展
IF 2.5
3区 数学
Q1 MATHEMATICS, APPLIED
引用次数: 0
摘要
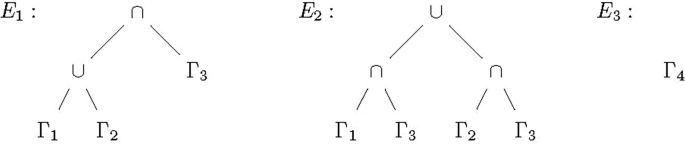
在投票理论和社会选择理论中,决策系统可以表示为简单的博弈,即通过参与者或选民及其获胜联盟集定义的合作博弈。加权投票博弈形成了简单博弈的一个众所周知的严格子类,其中每个玩家都有一个投票权重,因此如果其成员的权重总和超过给定的配额,则联盟获胜。由于获胜联盟的数量与玩家数量呈指数关系,所以简单的游戏可以更简洁地表示为加权投票游戏的交叉点或联盟。一个简单游戏的维度(协维)是加权投票游戏的最小数量,这样它们的交集(联合)就是给定的游戏。众所周知,存在具有高(co)维数的投票系统。本文引入了多维度作为加权投票博弈中具有交集和联合的表达式的最小尺寸,以获得所考虑的简单博弈。我们将这一概念推广到加权投票博弈的子类,并分析了这些子类的生成性质。我们还描述了在没有虚拟参与者的加权投票博弈集上具有有限广义多维度的简单博弈。我们提供了一个全面的分类,简单的游戏,直到一定数量的玩家。这些结果补充了广义(co)维的类似分类结果。我们的结果显示了广义多维度如何允许更简单、更紧凑地表示游戏,甚至对于少量玩家和子类也是如此。本文章由计算机程序翻译,如有差异,请以英文原文为准。
Multidimension: a dimensionality extension of simple games
Abstract In voting theory and social choice theory, decision systems can be represented as simple games, i.e., cooperative games defined through their players or voters and their set of winning coalitions. The weighted voting games form a well-known strict subclass of simple games, where each player has a voting weight so that a coalition wins if the sum of weights of their members exceeds a given quota. Since the number of winning coalitions can be exponential in the number of players, simple games can be represented much more compactly as intersections or unions of weighted voting games. A simple game’s dimension (codimension) is the minimum number of weighted voting games such that their intersection (union) is the given game. It is known there are voting systems with a high (co)dimension. This work introduces the multidimension as the minimum size of an expression with intersections and unions on weighted voting games necessary to obtain the considered simple game. We generalize this notion to subclasses of weighted voting games and analyze the generative properties of these subclasses. We also characterize the simple games with finite generalized multidimension over the set of weighted voting games without dummy players. We provide a comprehensive classification for simple games up to a certain number of players. These results complement similar classification results for generalized (co)dimensions. Our results show how generalized multidimension allows representing more simple games and more compactly, even for a small number of players and for subclasses.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Computational & Applied Mathematics
Mathematics-Computational Mathematics
CiteScore
4.50
自引率
11.50%
发文量
352
审稿时长
>12 weeks
期刊介绍:
Computational & Applied Mathematics began to be published in 1981. This journal was conceived as the main scientific publication of SBMAC (Brazilian Society of Computational and Applied Mathematics).
The objective of the journal is the publication of original research in Applied and Computational Mathematics, with interfaces in Physics, Engineering, Chemistry, Biology, Operations Research, Statistics, Social Sciences and Economy. The journal has the usual quality standards of scientific international journals and we aim high level of contributions in terms of originality, depth and relevance.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


