Tateno反驳Bonato-Tardif、Thomasse和Tyomkyn猜想的一个例子

IF 0.4 4区数学 Q4 MATHEMATICS

Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg Pub Date : 2023-11-01 DOI:10.1007/s12188-023-00270-0

Davoud Abdi Kalow, Claude Laflamme, Atsushi Tateno, Robert Woodrow

{"title":"Tateno反驳Bonato-Tardif、Thomasse和Tyomkyn猜想的一个例子","authors":"Davoud Abdi Kalow, Claude Laflamme, Atsushi Tateno, Robert Woodrow","doi":"10.1007/s12188-023-00270-0","DOIUrl":null,"url":null,"abstract":"<div><p>In his 2008 thesis [16] , Tateno claimed a counterexample to the Bonato–Tardif conjecture regarding the number of equimorphy classes of trees. In this paper we revisit Tateno’s unpublished ideas to provide a rigorous exposition, constructing locally finite trees having an arbitrary finite number of equimorphy classes; an adaptation provides partial orders with a similar conclusion. At the same time these examples also disprove conjectures by Thomassé and Tyomkyn.\n</p></div>","PeriodicalId":50932,"journal":{"name":"Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg","volume":null,"pages":null},"PeriodicalIF":0.4000,"publicationDate":"2023-11-01","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"6","resultStr":"{\"title\":\"An example of Tateno disproving conjectures of Bonato–Tardif, Thomasse, and Tyomkyn\",\"authors\":\"Davoud Abdi Kalow, Claude Laflamme, Atsushi Tateno, Robert Woodrow\",\"doi\":\"10.1007/s12188-023-00270-0\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<div><p>In his 2008 thesis [16] , Tateno claimed a counterexample to the Bonato–Tardif conjecture regarding the number of equimorphy classes of trees. In this paper we revisit Tateno’s unpublished ideas to provide a rigorous exposition, constructing locally finite trees having an arbitrary finite number of equimorphy classes; an adaptation provides partial orders with a similar conclusion. At the same time these examples also disprove conjectures by Thomassé and Tyomkyn.\\n</p></div>\",\"PeriodicalId\":50932,\"journal\":{\"name\":\"Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg\",\"volume\":null,\"pages\":null},\"PeriodicalIF\":0.4000,\"publicationDate\":\"2023-11-01\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"6\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg\",\"FirstCategoryId\":\"100\",\"ListUrlMain\":\"https://link.springer.com/article/10.1007/s12188-023-00270-0\",\"RegionNum\":4,\"RegionCategory\":\"数学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q4\",\"JCRName\":\"MATHEMATICS\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg","FirstCategoryId":"100","ListUrlMain":"https://link.springer.com/article/10.1007/s12188-023-00270-0","RegionNum":4,"RegionCategory":"数学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q4","JCRName":"MATHEMATICS","Score":null,"Total":0}

引用次数: 6

摘要

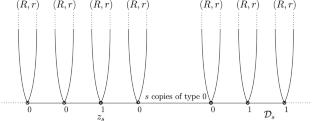
在他2008年的论文[16]中，Tateno提出了Bonato-Tardif猜想关于树的等对称类数量的反例。在本文中，我们回顾了Tateno未发表的思想，以提供一个严格的解释，构造具有任意有限数量的等价类的局部有限树;改编提供了具有类似结论的部分顺序。与此同时，这些例子也反驳了thomass和Tyomkyn的猜想。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

An example of Tateno disproving conjectures of Bonato–Tardif, Thomasse, and Tyomkyn

查看原文本刊更多论文

An example of Tateno disproving conjectures of Bonato–Tardif, Thomasse, and Tyomkyn

In his 2008 thesis [16] , Tateno claimed a counterexample to the Bonato–Tardif conjecture regarding the number of equimorphy classes of trees. In this paper we revisit Tateno’s unpublished ideas to provide a rigorous exposition, constructing locally finite trees having an arbitrary finite number of equimorphy classes; an adaptation provides partial orders with a similar conclusion. At the same time these examples also disprove conjectures by Thomassé and Tyomkyn.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg 数学-数学

CiteScore

0.80

自引率

0.00%

发文量

审稿时长

>12 weeks

期刊介绍： The first issue of the "Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg" was published in the year 1921. This international mathematical journal has since then provided a forum for significant research contributions. The journal covers all central areas of pure mathematics, such as algebra, complex analysis and geometry, differential geometry and global analysis, graph theory and discrete mathematics, Lie theory, number theory, and algebraic topology.