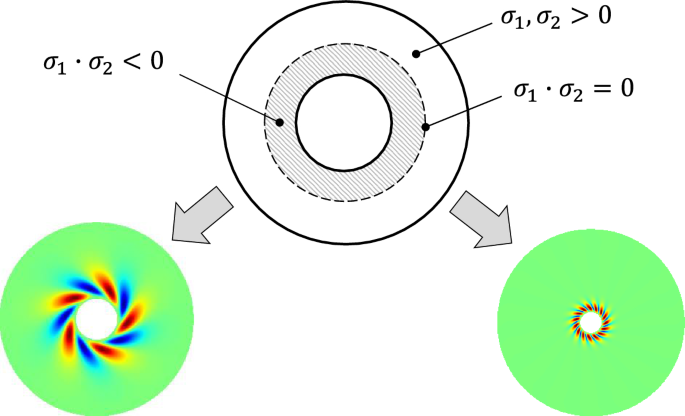
Shear-induced wrinkling in accelerating thin elastic discs

Abstract The wrinkling instabilities produced by in-plane angular accelerations in a rotating disc are discussed here in a particular limit of relevance to very thin plates. By coupling the classical linear elasticity solution for this configuration with the Föppl–von Kármán plate buckling equation, a fourth-order boundary-value problem with variable coefficients is obtained. The singular-perturbation character of the resulting problem arises from a combination of factors encompassing both the pre-stress (due to the spinning motion) and the geometry of the annular domain. With the help of a simplified multiple-scale perturbation method in conjunction with matched asymptotics, we succeed in capturing the dependence of the critical (wrinkling) acceleration on the instantaneous speed of the disc as well as other physical parameters. We show that the asymptotic predictions compare well with the results of direct numerical simulations of the original bifurcation problem. The limitations of the formulae obtained are also considered, and some practical suggestions for improving their accuracy are suggested.