Morelli-Włodarczyk协同主义和屋顶翻转的例子
IF 0.5
2区 数学
Q2 MATHEMATICS
引用次数: 0
摘要
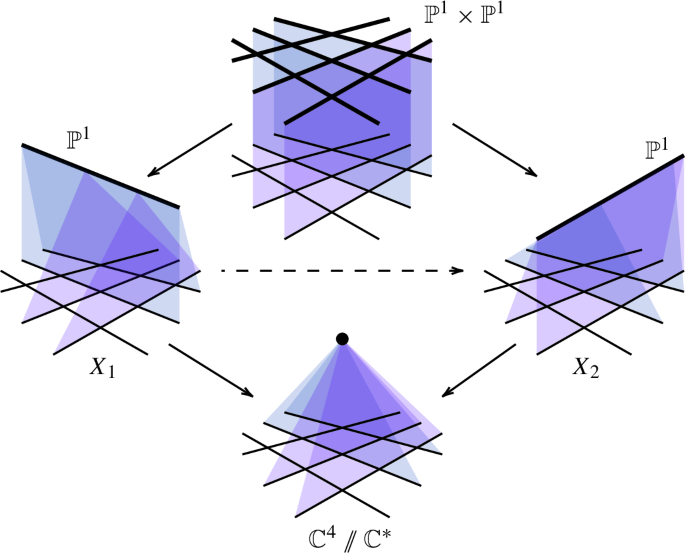
摘要引入了屋顶翻转的概念,即普通射影变体中的一种小修正,它是由包含两个射影束结构的Picard数2的光滑射影变体来建模的。例子包括Atiyah触发器和Mukai触发器,分别由$$\mathbb {P}^1\times \mathbb {P}^1$$ p1 × p1和$$\mathbb {P}\left( T_{\mathbb {P}^2}\right) $$ P T p2建模。利用Morelli-Włodarczyk配合定理,我们证明了任何具有$${\mathbb C}^*$$ C * -作用且只有两个不动点分量的Picard数1的光滑投影变数都能引起屋顶翻转。本文章由计算机程序翻译,如有差异,请以英文原文为准。
Morelli-Włodarczyk cobordism and examples of rooftop flips
Abstract We introduce the notion of rooftop flip, namely a small modification among normal projective varieties which is modeled by a smooth projective variety of Picard number 2 admitting two projective bundle structures. Examples include the Atiyah flop and the Mukai flop, modeled respectively by $$\mathbb {P}^1\times \mathbb {P}^1$$ P 1 × P 1 and by $$\mathbb {P}\left( T_{\mathbb {P}^2}\right) $$ P T P 2 . Using the Morelli-Włodarczyk cobordism, we prove that any smooth projective variety of Picard number 1, endowed with a $${\mathbb C}^*$$ C ∗ -action with only two fixed point components, induces a rooftop flip.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Collectanea Mathematica
数学-数学
CiteScore
2.70
自引率
9.10%
发文量
36
审稿时长
>12 weeks
期刊介绍:
Collectanea Mathematica publishes original research peer reviewed papers of high quality in all fields of pure and applied mathematics. It is an international journal of the University of Barcelona and the oldest mathematical journal in Spain. It was founded in 1948 by José M. Orts. Previously self-published by the Institut de Matemàtica (IMUB) of the Universitat de Barcelona, as of 2011 it is published by Springer.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


