Numerical Simulation of a Turbulent Gas Flow over a Wavy Surface
IF 0.58
Q3 Engineering
引用次数: 0
Abstract
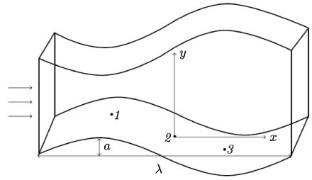
We use the OpnFoam package to numerically study the turbulent flow over a wavy surface for various values of the amplitude and wavelength of the perturbation of the channel wall. The RANS and LES models are used to describe turbulent characteristics. The Reynolds number in the flow is 20 000. The average profiles of velocities and shear stresses on the channel wall are obtained. The values of the amplitude and phase shift for perturbations of the shear stress are calculated for various geometrical parameters of the channel. Comparison with the theoretical model and experimental results is carried out.
波浪表面湍流气体流动的数值模拟
我们使用OpnFoampackage对波浪形表面上的湍流流动进行了数值研究,研究了不同波浪形表面上通道壁扰动的振幅和波长。RANS和LES模型用于描述湍流特性。流动中的雷诺数为20000。得到了通道壁上速度和剪应力的平均分布。计算了各种通道几何参数下剪切应力扰动的幅值和相移值。并与理论模型和实验结果进行了比较。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Journal of Applied and Industrial Mathematics
Engineering-Industrial and Manufacturing Engineering
CiteScore
1.00
自引率
0.00%
发文量
16
期刊介绍:
Journal of Applied and Industrial Mathematics is a journal that publishes original and review articles containing theoretical results and those of interest for applications in various branches of industry. The journal topics include the qualitative theory of differential equations in application to mechanics, physics, chemistry, biology, technical and natural processes; mathematical modeling in mechanics, physics, engineering, chemistry, biology, ecology, medicine, etc.; control theory; discrete optimization; discrete structures and extremum problems; combinatorics; control and reliability of discrete circuits; mathematical programming; mathematical models and methods for making optimal decisions; models of theory of scheduling, location and replacement of equipment; modeling the control processes; development and analysis of algorithms; synthesis and complexity of control systems; automata theory; graph theory; game theory and its applications; coding theory; scheduling theory; and theory of circuits.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


