Blending type approximation by G B S
IF 1.6
3区 数学
Q1 Mathematics
Journal of Inequalities and Applications
Pub Date : 2018-01-01
Epub Date: 2018-10-01
DOI:10.1186/s13660-018-1862-0
引用次数: 9
Abstract
In this paper, we introduce a family of operators of bivariate tensor product of λ-Bernstein-Kantorovich type. We estimate the rate of convergence of such operators for B-continuous and B-differentiable functions by using the mixed modulus of smoothness, establish the Voronovskaja type asymptotic formula for the bivariate λ-Bernstein-Kantorovich operators, as well as give some examples and their graphs to show the effect of convergence.
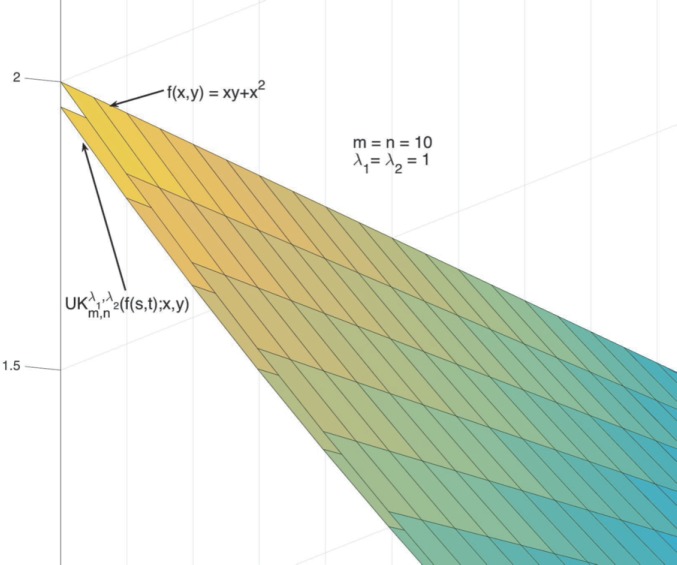
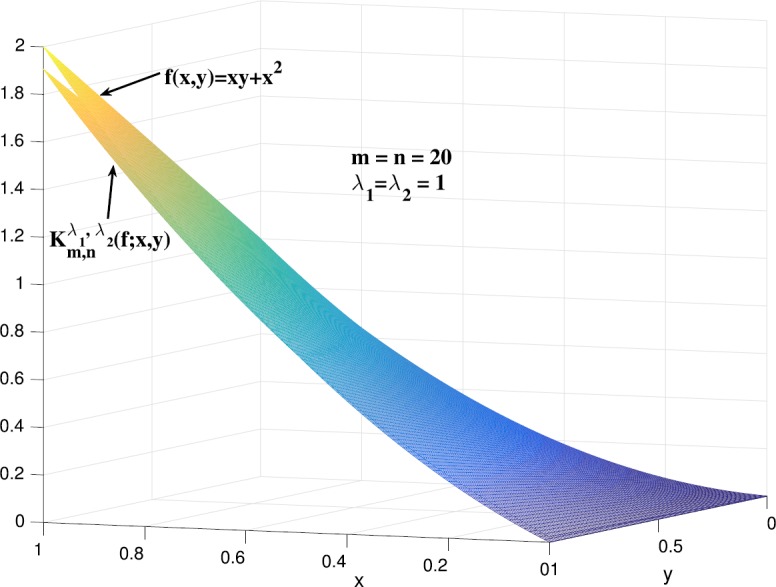
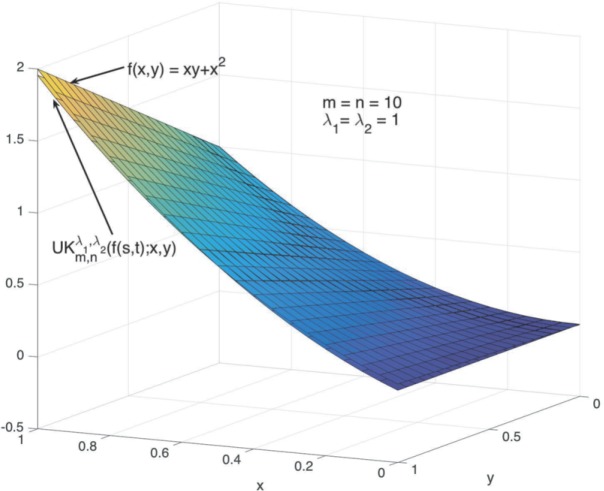
λ-Bernstein-Kantorovich型二元张量积的G -B - S算子的混合型逼近。
本文引入了λ-Bernstein-Kantorovich型二元张量积的G B S算子族。利用光滑的混合模估计了b -连续函数和b -可微函数的这类算子的收敛速度,建立了二元λ-Bernstein-Kantorovich算子的Voronovskaja型渐近公式,并给出了一些例子和图来说明收敛效果。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Journal of Inequalities and Applications
MATHEMATICS, APPLIED-MATHEMATICS
CiteScore
3.30
自引率
6.20%
发文量
136
审稿时长
3 months
期刊介绍:
The aim of this journal is to provide a multi-disciplinary forum of discussion in mathematics and its applications in which the essentiality of inequalities is highlighted. This Journal accepts high quality articles containing original research results and survey articles of exceptional merit. Subject matters should be strongly related to inequalities, such as, but not restricted to, the following: inequalities in analysis, inequalities in approximation theory, inequalities in combinatorics, inequalities in economics, inequalities in geometry, inequalities in mechanics, inequalities in optimization, inequalities in stochastic analysis and applications.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


