Regular logarithmic connections.
IF 1.4
2区 数学
Q1 MATHEMATICS
引用次数: 0
Abstract
We introduce the notion of a regular integrable connection on a smooth log scheme over and construct an equivalence between the category of such connections and the category of integrable connections on its analytification, compatible with de Rham cohomology. This extends the work of Deligne (when the log structure is trivial), and combined with the work of Ogus yields a topological description of the category of regular connections in terms of certain constructible sheaves on the Kato-Nakayama space. The key ingredients are the notion of a canonical extension in this context and the existence of good compactifications of log schemes obtained recently by Włodarczyk.
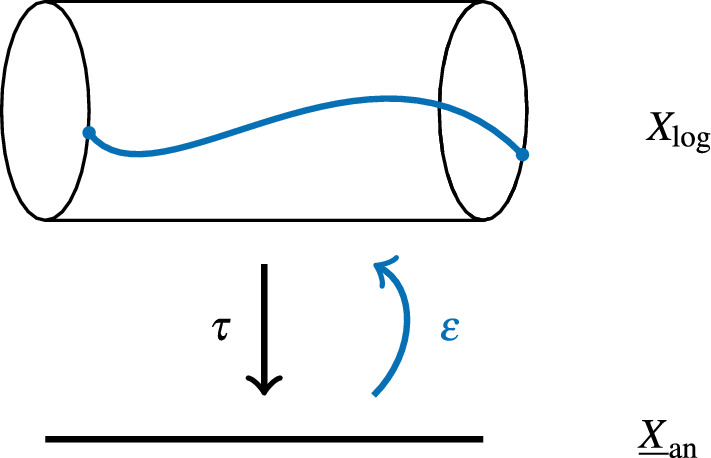
正则对数连接。
我们引入了C上光滑对数格式上正则可积连接的概念,并构造了这类连接的范畴与可积连接的范畴在其分析上的等价,与de Rham上同调相容。这扩展了Deligne的工作(当日志结构是平凡的),并与Ogus的工作相结合,产生了关于在Kato-Nakayama空间上的某些可构造轴的规则连接类别的拓扑描述。在这种情况下,关键因素是规范扩展的概念,以及最近通过Włodarczyk获得的对数格式的良好紧化。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Mathematische Annalen
数学-数学
CiteScore
2.90
自引率
7.10%
发文量
181
审稿时长
4-8 weeks
期刊介绍:
Begründet 1868 durch Alfred Clebsch und Carl Neumann. Fortgeführt durch Felix Klein, David Hilbert, Otto Blumenthal, Erich Hecke, Heinrich Behnke, Hans Grauert, Heinz Bauer, Herbert Amann, Jean-Pierre Bourguignon, Wolfgang Lück und Nigel Hitchin.
The journal Mathematische Annalen was founded in 1868 by Alfred Clebsch and Carl Neumann. It was continued by Felix Klein, David Hilbert, Otto Blumenthal, Erich Hecke, Heinrich Behnke, Hans Grauert, Heinz Bauer, Herbert Amann, Jean-Pierre Bourguigon, Wolfgang Lück and Nigel Hitchin.
Since 1868 the name Mathematische Annalen stands for a long tradition and high quality in the publication of mathematical research articles. Mathematische Annalen is designed not as a specialized journal but covers a wide spectrum of modern mathematics.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


