Loschmidt echo for deformed Wigner matrices
IF 1.4
3区 物理与天体物理
Q3 PHYSICS, MATHEMATICAL
引用次数: 0
Abstract
We consider two Hamiltonians that are close to each other, \(H_1 \approx H_2 \), and analyze the time decay of the corresponding Loschmidt echo \(\mathfrak {M}(t):= |\langle \psi _0, \textrm{e}^{\textrm{i} t H_2} \textrm{e}^{-\textrm{i} t H_1} \psi _0 \rangle |^2\) that expresses the effect of an imperfect time reversal on the initial state \(\psi _0\). Our model Hamiltonians are deformed Wigner matrices that do not share a common eigenbasis. The main tools are new two-resolvent laws for such \(H_1\) and \(H_2\).
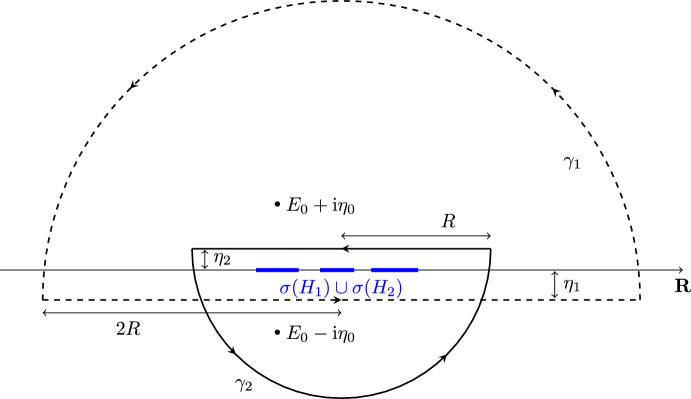
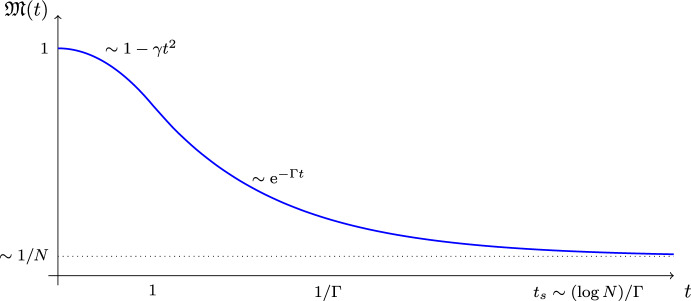

变形Wigner矩阵的Loschmidt回波。
我们考虑两个彼此接近的哈密顿量,H 1≈H 2,并分析相应的洛施密特回波M (t)的时间衰减:= |⟨ψ 0, e it H 2 e - i i H 1 ψ 0⟩| 2,它表示不完全时间反转对初始状态ψ 0的影响。我们的模型哈密顿矩阵是不具有共同特征基的变形维格纳矩阵。主要的工具是关于h1和h2的新的双分解定律。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Letters in Mathematical Physics
物理-物理:数学物理
CiteScore
2.40
自引率
8.30%
发文量
111
审稿时长
3 months
期刊介绍:
The aim of Letters in Mathematical Physics is to attract the community''s attention on important and original developments in the area of mathematical physics and contemporary theoretical physics. The journal publishes letters and longer research articles, occasionally also articles containing topical reviews. We are committed to both fast publication and careful refereeing. In addition, the journal offers important contributions to modern mathematics in fields which have a potential physical application, and important developments in theoretical physics which have potential mathematical impact.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


