A basic system of paraconsistent Nelsonian logic of conditionals.
IF 0.6
3区 数学
Q4 COMPUTER SCIENCE, ARTIFICIAL INTELLIGENCE
Journal of Logic Language and Information
Pub Date : 2024-01-01
Epub Date: 2024-12-03
DOI:10.1007/s10849-024-09421-9
引用次数: 0
Abstract
We define a Kripke semantics for a conditional logic based on the propositional logic , the paraconsistent variant of Nelson's logic of strong negation; we axiomatize the minimal system induced by this semantics. The resulting logic, which we call , shows strong connections both with the basic intuitionistic logic of conditionals introduced earlier in (Olkhovikov, 2023) and with the -based modal logic introduced in (Odintsov and Wansing, 2004) as one of the possible counterparts to the classical modal system . We map these connections by looking into the embeddings which obtain between the aforementioned systems.
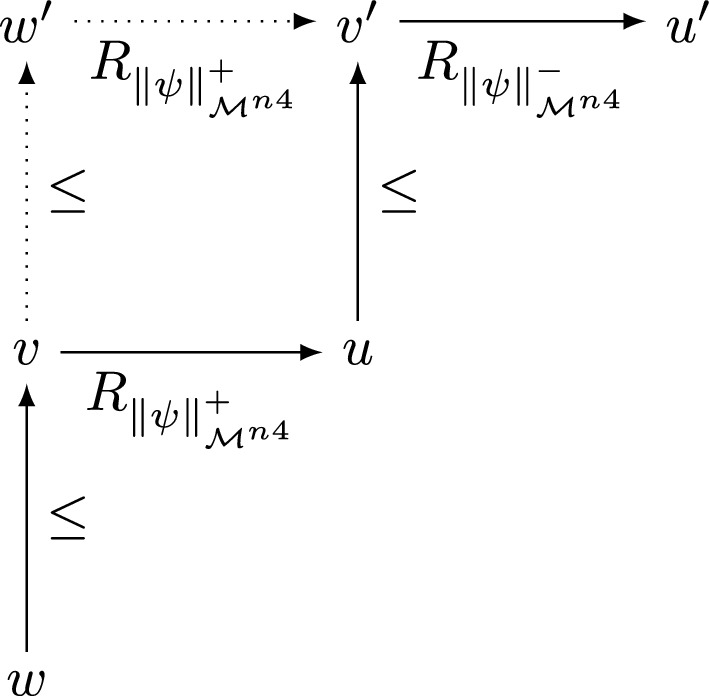
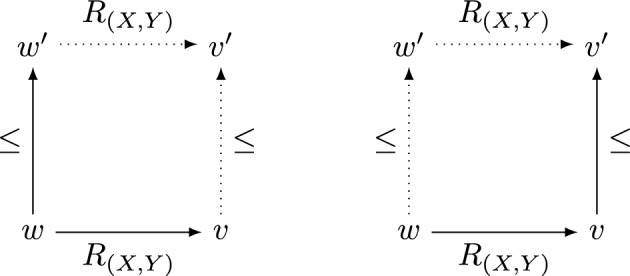
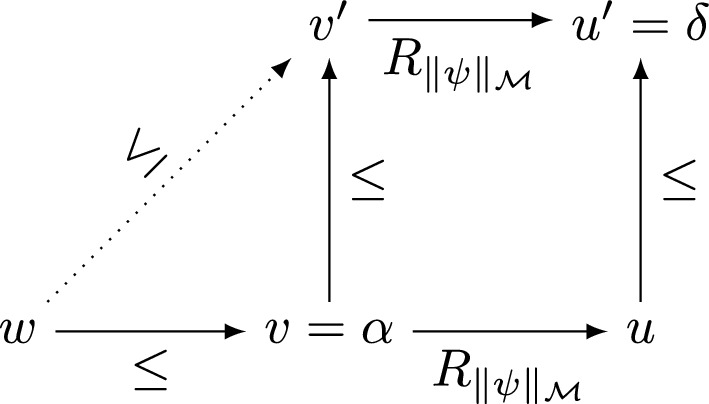
一个基本的副一致尼尔森条件逻辑系统。
我们定义了基于命题逻辑n4的条件逻辑的Kripke语义,这是Nelson的强否定逻辑的副一致变体;我们公理化了由这个语义引起的最小系统。由此产生的逻辑,我们称之为n4ck,与之前在(Olkhovikov, 2023)中引入的IntCK的基本直觉主义条件逻辑以及(Odintsov和Wansing, 2004)中引入的基于n4的模态逻辑FSK d(作为经典模态系统K的可能对应物之一)具有很强的联系。我们通过查看上述系统之间获得的嵌入来映射这些连接。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Journal of Logic Language and Information
COMPUTER SCIENCE, ARTIFICIAL INTELLIGENCEL-LOGIC
CiteScore
1.70
自引率
12.50%
发文量
40
期刊介绍:
The scope of the journal is the logical and computational foundations of natural, formal, and programming languages, as well as the different forms of human and mechanized inference. It covers the logical, linguistic, and information-theoretic parts of the cognitive sciences.
Examples of main subareas are Intentional Logics including Dynamic Logic; Nonmonotonic Logic and Belief Revision; Constructive Logics; Complexity Issues in Logic and Linguistics; Theoretical Problems of Logic Programming and Resolution; Categorial Grammar and Type Theory; Generalized Quantification; Information-Oriented Theories of Semantic Structure like Situation Semantics, Discourse Representation Theory, and Dynamic Semantics; Connectionist Models of Logical and Linguistic Structures. The emphasis is on the theoretical aspects of these areas.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


