On the atomistic origin of internal length scale in strain-gradient plasticity models: The case of grain boundary structures and energies
IF 8.3
1区 材料科学
Q1 MATERIALS SCIENCE, MULTIDISCIPLINARY
引用次数: 0
Abstract
The mechanical behavior of polycrystalline materials is controlled by microstructural size effects such as grain size or precipitate size. Various models of strain gradient plasticity have been proposed to capture such size effects, many of which have incorporated geometrically-necessary dislocation (GND) densities to introduce characteristic internal lengths. Recent developments have focused on models that incorporate a GND density into the internal energy functional. In such models, one needs to physically justify the functional form chosen and quantify the inherent internal length parameter. Our present study aims at probing relevant forms and internal length values in the case of grain boundary (GB) atomistic structures and core energies. We use an atomistic-to-continuum crossover approach that predicts an atomistic structure dependent GB energy by molecular static simulations, which is then recovered at the continuum-level by using a strain gradient, atomistically informed, field dislocation mechanics fast Fourier transform model. This allows (i) delineating the atomistic structure of GBs using an equivalent Nye GND density, and (ii) capturing the associated continuous elastic fields in the GB core area. We probe (i) a generalized non-quadratic GND density dependent energy functional to account for the core energy of defects, and (ii) elucidate the contributions of core versus elastic energy to the overall GB excess energy. We investigate and discuss the possible relevant choices for the energy functional form, as well as the physical origin of the inherent internal length parameter and its dependence to the types of grain boundaries, atomistic structures, and spatial resolution.
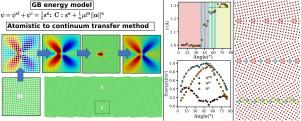
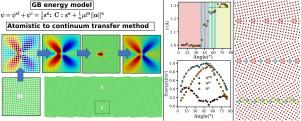
应变梯度塑性模型中内部长度尺度的原子论起源:晶界结构与能量
多晶材料的机械行为受微结构尺寸效应(如晶粒尺寸或沉淀尺寸)的控制。为了捕捉这种尺寸效应,人们提出了各种应变梯度塑性模型,其中许多模型都加入了几何必要位错(GND)密度,以引入特征内长。最近的发展集中于将 GND 密度纳入内能函数的模型。在这些模型中,我们需要从物理上证明所选择的函数形式,并量化固有的内部长度参数。本研究旨在探究晶界(GB)原子结构和核心能量的相关形式和内部长度值。我们采用原子到连续的交叉方法,通过分子静态模拟预测与原子结构相关的 GB 能量,然后使用应变梯度、原子信息、场位错力学快速傅立叶变换模型在连续层面恢复该能量。这样就能(i)使用等效奈伊 GND 密度来划分 GB 的原子结构,(ii)捕捉 GB 核心区域的相关连续弹性场。我们探究了(i) 与 GND 密度相关的广义非二次能量函数,以解释缺陷的核心能量,(ii) 阐明核心能量和弹性能量对整个 GB 过剩能量的贡献。我们研究并讨论了能量函数形式的可能相关选择,以及固有内部长度参数的物理来源及其与晶界类型、原子结构和空间分辨率的关系。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Acta Materialia
工程技术-材料科学:综合
CiteScore
16.10
自引率
8.50%
发文量
801
审稿时长
53 days
期刊介绍:
Acta Materialia serves as a platform for publishing full-length, original papers and commissioned overviews that contribute to a profound understanding of the correlation between the processing, structure, and properties of inorganic materials. The journal seeks papers with high impact potential or those that significantly propel the field forward. The scope includes the atomic and molecular arrangements, chemical and electronic structures, and microstructure of materials, focusing on their mechanical or functional behavior across all length scales, including nanostructures.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


