Effects of Modified Potential and Quantum Correction in the Generalized Perturbed Interacting CR3BP with Variable Mass

IF 0.6 4区物理与天体物理 Q4 ASTRONOMY & ASTROPHYSICS

Solar System Research Pub Date : 2024-10-15 DOI:10.1134/S0038094624601385

Abdullah, Majhar Ali

引用次数: 0

Abstract

The aim of this paper is to investigate the motion properties of the infinitesimal body under the effects of gravitational forces, the quantum correction, variable mass, modified Newtonian force, interaction between bodies, triaxial parameters, Coriolis and centrifugal forces in the restricted three-body configuration. Using Jeans law, Meshcherskii space-time transformations and the above perturbations, we evaluate the equations of motion and quasi-Jacobian integral. And then, we numerically illustrate the locations of equilibrium points, regions of motion, Poincare surfaces of section, basins of attraction, periodic orbits and stability of the equilibrium points.

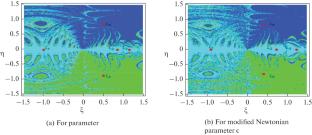
Abstract Image

查看原文本刊更多论文

质量可变的广义扰动相互作用 CR3BP 中的修正势能和量子修正效应

本文旨在研究受限三体构型中无穷小体在引力、量子修正、变质量、修正牛顿力、体间相互作用、三轴参数、科里奥利力和离心力作用下的运动特性。利用杰恩斯定律、梅舍尔斯基时空变换和上述扰动，我们评估了运动方程和准雅各布积分。然后，我们用数值方法说明了平衡点的位置、运动区域、剖面波恩加尔曲面、吸引盆地、周期轨道和平衡点的稳定性。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Solar System Research 地学天文-天文与天体物理

CiteScore

1.60

自引率

33.30%

发文量

审稿时长

6-12 weeks

期刊介绍： Solar System Research publishes articles concerning the bodies of the Solar System, i.e., planets and their satellites, asteroids, comets, meteoric substances, and cosmic dust. The articles consider physics, dynamics and composition of these bodies, and techniques of their exploration. The journal addresses the problems of comparative planetology, physics of the planetary atmospheres and interiors, cosmochemistry, as well as planetary plasma environment and heliosphere, specifically those related to solar-planetary interactions. Attention is paid to studies of exoplanets and complex problems of the origin and evolution of planetary systems including the solar system, based on the results of astronomical observations, laboratory studies of meteorites, relevant theoretical approaches and mathematical modeling. Alongside with the original results of experimental and theoretical studies, the journal publishes scientific reviews in the field of planetary exploration, and notes on observational results.