Global Stability for Nonlinear Wave Equations Satisfying a Generalized Null Condition
IF 2.6
1区 数学
Q1 MATHEMATICS, APPLIED
引用次数: 0
Abstract
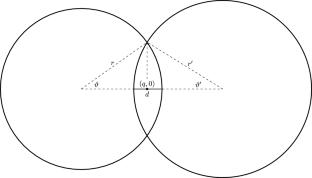
We prove global stability for nonlinear wave equations satisfying a generalized null condition. The generalized null condition is made to allow for null forms whose coefficients have bounded \(C^k\) norms. We prove both the pointwise decay and improved decay of good derivatives using bilinear energy estimates and duality arguments. Combining this strategy with the \(r^p\) estimates of Dafermos–Rodnianski then allows us to prove the global stability. The proof requires analyzing the geometry of intersecting null hypersurfaces adapted to solutions of wave equations.
满足广义零条件的非线性波方程的全局稳定性
我们证明了满足广义空条件的非线性波方程的全局稳定性。广义空条件允许系数具有有界 \(C^k\) 规范的空形式。我们利用双线性能量估计和对偶论证证明了好导数的点式衰减和改进衰减。将这一策略与 Dafermos-Rodnianski 的 \(r^p\) 估计相结合,我们就能证明全局稳定性。证明需要分析与波方程解相适应的相交空超曲面的几何。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊
CiteScore
5.10
自引率
8.00%
发文量
98
审稿时长
4-8 weeks
期刊介绍:
The Archive for Rational Mechanics and Analysis nourishes the discipline of mechanics as a deductive, mathematical science in the classical tradition and promotes analysis, particularly in the context of application. Its purpose is to give rapid and full publication to research of exceptional moment, depth and permanence.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


