Pairwise balancing of forces in traveling granular waves
IF 2.7
3区 数学
Q1 MATHEMATICS, APPLIED
引用次数: 0
Abstract
A survey is given of the forces at work inside traveling roll waves and monoclinal flood waves in dry granular matter. By numerically solving the generalized Saint-Venant equations for shallow granular flow, we find a hierarchy of pairwise near-balancing forces, which together are responsible for sustaining the shape of the nonlinear waves in question. The leading force pair is gravity vs. friction, followed by inertia vs. pressure, while the minor imbalance left by the aforementioned pairs is settled by a (small though essential) diffusive force.
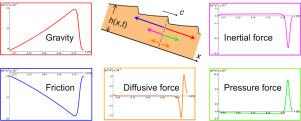
行进粒状波中的成对平衡力
本文探讨了在干燥颗粒物质中行进的滚动波和单向洪波内部的作用力。通过数值求解浅层粒状流的广义圣-维南方程,我们发现了一对接近平衡的力的层次结构,它们共同负责维持有关非线性波的形状。最主要的力对是重力与摩擦力,其次是惯性力与压力,而上述力对留下的微小不平衡则由扩散力(虽然很小,但至关重要)来解决。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Physica D: Nonlinear Phenomena
物理-物理:数学物理
CiteScore
7.30
自引率
7.50%
发文量
213
审稿时长
65 days
期刊介绍:
Physica D (Nonlinear Phenomena) publishes research and review articles reporting on experimental and theoretical works, techniques and ideas that advance the understanding of nonlinear phenomena. Topics encompass wave motion in physical, chemical and biological systems; physical or biological phenomena governed by nonlinear field equations, including hydrodynamics and turbulence; pattern formation and cooperative phenomena; instability, bifurcations, chaos, and space-time disorder; integrable/Hamiltonian systems; asymptotic analysis and, more generally, mathematical methods for nonlinear systems.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


