Nonequilibrium Transition between Dissipative Time Crystals
引用次数: 0
Abstract
We show a dissipative phase transition in a driven nonlinear quantum oscillator in which a discrete time-translation symmetry is spontaneously broken in two different ways. The corresponding regimes display either discrete or incommensurate time-crystal order, which we analyze numerically and analytically beyond the classical limit, addressing observable dynamics, phenomenology in different (laboratory and rotating) frames, Liouvillian spectral features, and quantum fluctuations. Via an effective semiclassical description, we show that phase diffusion dominates in the incommensurate time crystal (or continuous time crystal in the rotating frame), which manifests as a band of eigenmodes with a lifetime growing linearly with the mean-field excitation number. Instead, in the discrete time-crystal phase, the leading fluctuation process corresponds to quantum activation with a single mode that has an exponentially growing lifetime. Interestingly, the transition between these two regimes manifests itself already in the quantum regime as a spectral singularity, namely, as an exceptional point mediating between phase diffusion and quantum activation. Finally, we discuss this transition between different time-crystal orders in the context of synchronization phenomena.
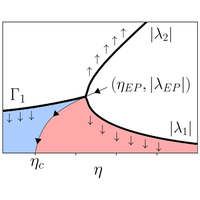
耗散时间晶体之间的非平衡转变
我们展示了驱动非线性量子振荡器中的耗散相变,其中离散时间平移对称性以两种不同方式自发被打破。我们对其进行了超越经典极限的数值和分析,探讨了可观测的动力学、不同(实验室和旋转)框架下的现象学、Liouvillian 光谱特征和量子波动。通过有效的半经典描述,我们表明相位扩散在不相称时间晶体(或旋转框架中的连续时间晶体)中占主导地位,表现为寿命与平均场激励数线性增长的特征模带。相反,在离散时间晶体阶段,前导波动过程对应于量子激活,单个模式的寿命呈指数增长。有趣的是,这两种体系之间的过渡在量子体系中已经表现为光谱奇点,即介于相扩散和量子激活之间的特殊点。最后,我们结合同步现象讨论了不同时晶阶之间的过渡。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


