Analytical solutions for long-time steady state Boussinesq gravity currents flowing along a horizontal boundary of finite length

Abstract

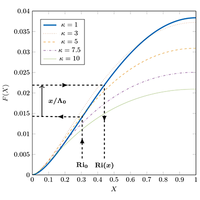
This paper presents analytical solutions for a steady turbulent miscible gravity current flowing along a horizontal rigid boundary of finite length into a quiescent uniform environment. These solutions are obtained from the governing equations (mass, momentum, and buoyancy) originally proposed by Ellison and Turner [J. Fluid Mech. 6, 423 (1959)] for a buoyant layer of fluid in the Boussinesq approximation. For a constant drag coefficient $C_d$ and the specific entrainment law $E\propto {\text{Ri}}^{-1}, \text{Ri}$ being the local Richardson number, we first derived a system of coupled ordinary differential equations describing the longitudinal evolution of the velocity $u$, the height $h$, the density deficit $\eta$, and the Richardson number $\text{Ri}$ of the current. For an initially supercritical flow $\left({\text{Ri}}_0<1\right)$, explicit relations are found for $u\left(x\right), h\left(x\right),$ and $\eta \left(x\right)$ solely as a function of the Richardson number $\text{Ri}\left(x\right)$. The longitudinal evolution of the Richardson number is then theoretically obtained from a universal function $F$ which can be tabulated and, as in the present paper, also plotted. The function $F$ allows us to determine (and only from the knowledge of the boundary conditions at the source) whether the flow remains supercritical over the whole length of the rigid boundary, or might transit towards a subcritical state ($\text{Ri}>1$). In this latter case, the mathematical resolution is modified by including a discontinuity similar to a hydraulic jump. The location and amplitude of this discontinuity are calculated from an additional universal function $G$ and the injection conditions. The method is finally extended to provide analytical solutions for other classical entrainment laws.