Critical charges for droplet collisions
IF 2.5
3区 物理与天体物理
Q2 PHYSICS, FLUIDS & PLASMAS
引用次数: 0
Abstract
Two micron-sized water droplets approaching each other do not always coalesce due to the cushioning effect of the air between them. When the droplets do not carry any electrical charges, one needs to consider the breakdown of hydrodynamics at very small scales to decide whether the droplets collide and coalesce or not. In contrast, two approaching droplets that are oppositely charged always coalesce if the charges are large enough. To find the charge for which the transition to charge-dominated collisions occurs, we computed the collision efficiency of charged, hydrodynamically interacting droplets settling in quiescent air, including the noncontinuum regime at small interfacial distances. For oppositely charged droplets, we find that the transition occurs when a saddle point of the relative droplet dynamics exits the region within which the continuum hydrodynamics breaks down. For cloud droplets with radii 16 and , we observe that the transition occurs at elementary charges . For charges smaller than this, we predict that the coalescence rate depends primarily upon the Knudsen number (, the ratio of the mean-free-path of air to the mean droplet radius), whereas coalescence for much larger charges does not depend upon . For droplets charged with the same polarity, we find the critical charge to be substantially larger ( for the above radii) for reasons that we discuss.
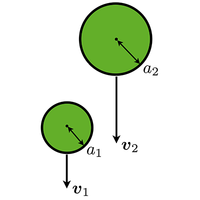
液滴碰撞的临界电荷
两个微米大小的水滴相互靠近时,由于它们之间空气的缓冲作用,并不总是能够凝聚在一起。当水滴不带任何电荷时,我们需要考虑流体力学在极小尺度上的破坏,以决定水滴是否碰撞和凝聚。相反,如果电荷足够大,两个接近的带相反电荷的液滴总是会凝聚在一起。为了找到过渡到电荷主导碰撞的电荷,我们计算了静态空气中沉降的带电流体力学相互作用液滴的碰撞效率,包括小界面距离的非连续状态。对于带相反电荷的液滴,我们发现当相对液滴动力学的鞍点离开连续流体力学破裂的区域时,就发生了转变。对于半径为 16µm 和 20µm 的云滴,我们观察到过渡发生在 ∼103 基本电荷 e 时。对于小于此值的电荷,我们预测凝聚率主要取决于 Knudsen 数(Kn,空气平均自由路径与平均液滴半径之比),而对于大得多的电荷,凝聚则不取决于 Kn。对于带相同极性电荷的液滴,我们发现临界电荷要大得多(对于上述半径,临界电荷为 ∼104e),原因我们将在下文讨论。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊
Physical Review Fluids
Chemical Engineering-Fluid Flow and Transfer Processes
CiteScore
5.10
自引率
11.10%
发文量
488
期刊介绍:
Physical Review Fluids is APS’s newest online-only journal dedicated to publishing innovative research that will significantly advance the fundamental understanding of fluid dynamics. Physical Review Fluids expands the scope of the APS journals to include additional areas of fluid dynamics research, complements the existing Physical Review collection, and maintains the same quality and reputation that authors and subscribers expect from APS. The journal is published with the endorsement of the APS Division of Fluid Dynamics.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


