Non-thin rank jumps for double elliptic K3 surfaces
IF 0.6
4区 数学
Q3 MATHEMATICS
引用次数: 0
Abstract
For an elliptic surface \(\pi :X\rightarrow \mathbb {P}^1\) defined over a number field K, a theorem of Silverman shows that for all but finitely many fibres above K-rational points, the resulting elliptic curve over K has Mordell-Weil rank at least as large as the rank of the group of sections of \(\pi \). When X is a K3 surface with two distinct elliptic fibrations, we show that the set of K-rational points of \(\mathbb {P}^1\) for which this rank inequality is strict, is not a thin set, under certain hypothesis on the fibrations. Our results provide one of the first cases of this phenomenon beyond that of rational elliptic surfaces.
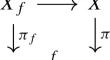
双椭圆 K3 曲面的非薄级跃迁
对于定义在数域 K 上的椭圆曲面 \(\pi :X\rightarrow \mathbb {P}^1\),西尔弗曼(Silverman)的一个定理表明,除了有限多个 K 有理点之上的纤维之外,K 上的椭圆曲线的莫德尔-韦尔阶(Mordell-Weil rank)至少与 \(\pi \)的截面群的阶一样大。当 X 是一个有两个不同椭圆纤分的 K3 曲面时,我们证明了在纤分的特定假设下,秩不等式严格的 \(\mathbb {P}^1\) 的 K 有理点集合不是一个薄集。我们的结果提供了这一现象在有理椭圆曲面之外的第一个案例。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Manuscripta Mathematica
数学-数学
CiteScore
1.40
自引率
0.00%
发文量
86
审稿时长
6-12 weeks
期刊介绍:
manuscripta mathematica was founded in 1969 to provide a forum for the rapid communication of advances in mathematical research. Edited by an international board whose members represent a wide spectrum of research interests, manuscripta mathematica is now recognized as a leading source of information on the latest mathematical results.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


