On the Infinite $$\varphi $$ -Order Solutions of Second Order Linear Differential Equations

IF 0.6 4区数学 Q3 MATHEMATICS

Computational Methods and Function Theory Pub Date : 2024-05-29 DOI:10.1007/s40315-024-00548-1

Hui Yu, Xiaomin Li

引用次数: 0

Abstract

In this paper, we consider the second order linear differential equation

where A, B and F with $B\not \equiv 0$ are entire functions. We find some appropriate conditions on A, B and F in terms of the $\varphi $-order which guarantee that every non-constant entire solution f of (†) has infinite $\varphi $-order, along with an additional relation between the hyper-$\varphi $-order of f and the $\varphi $-order of the dominating coefficient in (†).

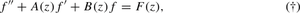
Abstract Image

查看原文本刊更多论文

论二阶线性微分方程的无限 $$\varphi $$ 阶解

在本文中，我们考虑了二阶线性微分方程，其中 A、B 和 F 都是$B\not \equiv 0$ 全函数。我们从 $\varphi $-阶的角度找到了一些关于 A、B 和 F 的适当条件，这些条件保证了 (†) 的每个非常数全解 f 具有无限的 $\varphi $-阶，同时还找到了 f 的超 $\varphi $-阶与 (†) 中支配系数的 $\varphi $-阶之间的附加关系。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Computational Methods and Function Theory MATHEMATICS, APPLIED-MATHEMATICS

CiteScore

3.20

自引率

0.00%

发文量

审稿时长

>12 weeks

期刊介绍： CMFT is an international mathematics journal which publishes carefully selected original research papers in complex analysis (in a broad sense), and on applications or computational methods related to complex analysis. Survey articles of high standard and current interest can be considered for publication as well.