Multiplicity of Normalized Solutions for Schrödinger Equations
IF 1.2
3区 数学
Q1 MATHEMATICS
Bulletin of the Malaysian Mathematical Sciences Society
Pub Date : 2024-05-27
DOI:10.1007/s40840-024-01713-4
引用次数: 0
Abstract
In this paper, we consider the following nonlinear Schrödinger equation with an \(L^2\)-constraint:
$$\begin{aligned} {\left\{ \begin{array}{ll} -\Delta u=\lambda u+\mu |u|^{q-2}u+|u|^{p-2}u \ \ \ \textrm{in}~\mathbb {R}^{N}, \\ \int _{\mathbb {R}^{N}}|u|^{2}dx=a^2, \ \ u\in H^1(\mathbb {R}^{N}), \end{array}\right. }\end{aligned}$$where \(N\ge 3\), \(a,\mu >0\), \(2<q<2+\frac{4}{N}<p<2^*\), \(2q+2N-pN<0\) and \(\lambda \in \mathbb {R}\) arises as a Lagrange multiplier. We deal with the concave and convex cases of energy functional constraints on the \(L^2\) sphere, and prove the existence of infinitely solutions with positive energy levels.
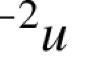
薛定谔方程归一化解的多重性
在本文中,我们考虑了以下具有(L^2\)约束条件的非线性薛定谔方程:$$\begin{aligned} {\left\{ \begin{array}{ll} -\Delta u=\lambda u+\mu |u|^{q-2}u+|u|^{p-2}u \\textrm{in}~\mathbb {R}^{N}、\int _{mathbb {R}^{N}}|u|^{2}dx=a^2, \ u\in H^1(\mathbb {R}^{N}), \end{array}\right.}end{aligned}$$ 其中(N\ge 3\),(a,\mu >0\),(2<q<2+frac{4}{N}<p<2^*\),(2q+2N-pN<0\)和(\lambda \in \mathbb {R}\)作为拉格朗日乘数出现。我们处理了能量函数约束在 \(L^2\) 球上的凹和凸情况,并证明了具有正能级的无限解的存在。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊
CiteScore
2.40
自引率
8.30%
发文量
176
审稿时长
3 months
期刊介绍:
This journal publishes original research articles and expository survey articles in all branches of mathematics. Recent issues have included articles on such topics as Spectral synthesis for the operator space projective tensor product of C*-algebras; Topological structures on LA-semigroups; Implicit iteration methods for variational inequalities in Banach spaces; and The Quarter-Sweep Geometric Mean method for solving second kind linear fredholm integral equations.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


