On Donkin's tilting module conjecture II: counterexamples
IF 1.4
1区 数学
Q1 MATHEMATICS
引用次数: 0
Abstract
In this paper we produce infinite families of counterexamples to Jantzen's question posed in 1980 on the existence of Weyl 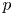 $p$-filtrations for Weyl modules for an algebraic group and Donkin's tilting module conjecture formulated in 1990. New techniques to exhibit explicit examples are provided along with methods to produce counterexamples in large rank from counterexamples in small rank. Counterexamples can be produced via our methods for all groups other than when the root system is of type
$p$-filtrations for Weyl modules for an algebraic group and Donkin's tilting module conjecture formulated in 1990. New techniques to exhibit explicit examples are provided along with methods to produce counterexamples in large rank from counterexamples in small rank. Counterexamples can be produced via our methods for all groups other than when the root system is of type  ${\rm A}_{n}$ or
${\rm A}_{n}$ or 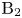 ${\rm B}_{2}$.
${\rm B}_{2}$.
关于唐金的倾斜模猜想 II:反例
在本文中,我们针对杨岑在 1980 年提出的关于代数群的 Weyl 模块是否存在 Weyl $p$ 滤波的问题,以及唐金在 1990 年提出的倾斜模块猜想,提出了无穷系列的反例。本书提供了展示明确例子的新技术,以及从小秩反例产生大秩反例的方法。除了根系统是 ${\rm A}_{n}$ 或 ${\rm B}_{2}$ 类型之外,所有群都可以通过我们的方法产生反例。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Compositio Mathematica
数学-数学
CiteScore
2.10
自引率
0.00%
发文量
62
审稿时长
6-12 weeks
期刊介绍:
Compositio Mathematica is a prestigious, well-established journal publishing first-class research papers that traditionally focus on the mainstream of pure mathematics. Compositio Mathematica has a broad scope which includes the fields of algebra, number theory, topology, algebraic and differential geometry and global analysis. Papers on other topics are welcome if they are of broad interest. All contributions are required to meet high standards of quality and originality. The Journal has an international editorial board reflected in the journal content.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


