CLT for \(\beta \)-Ensembles at High Temperature and for Integrable Systems: A Transfer Operator Approach
IF 1.3
3区 物理与天体物理
Q2 PHYSICS, MATHEMATICAL
引用次数: 0
Abstract
In this paper, we prove a polynomial central limit theorem for several integrable models and for the \(\beta \)-ensembles at high temperature with polynomial potential. Furthermore, we connect the mean values, the variances and the correlations of the moments of the Lax matrices of these integrable systems with the ones of the \(\beta \)-ensembles. Moreover, we show that the local functions’ space-correlations decay exponentially fast for the considered integrable systems. For these models, we also established a Berry–Esseen-type bound.
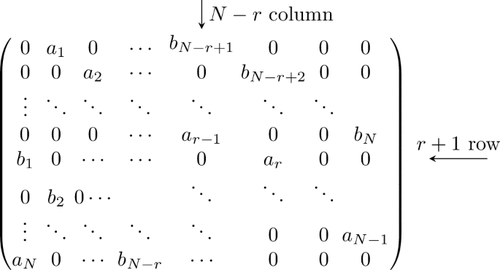
用于高温下 $$\beta $$ 组合和可积分系统的 CLT:转移算子方法
在本文中,我们证明了几种可积分模型的多项式中心极限定理,以及高温下具有多项式势的\(\beta \)-符号的多项式中心极限定理。此外,我们将这些可积分系统的 Lax 矩阵的均值、方差和相关性与 \(β\)-ensembles 矩阵的均值、方差和相关性联系起来。此外,我们还证明,对于所考虑的可积分系统,局部函数的空间相关性呈指数级快速衰减。对于这些模型,我们还建立了贝里-埃森型约束。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Annales Henri Poincaré
物理-物理:粒子与场物理
CiteScore
3.00
自引率
6.70%
发文量
108
审稿时长
6-12 weeks
期刊介绍:
The two journals Annales de l''Institut Henri Poincaré, physique théorique and Helvetica Physical Acta merged into a single new journal under the name Annales Henri Poincaré - A Journal of Theoretical and Mathematical Physics edited jointly by the Institut Henri Poincaré and by the Swiss Physical Society.
The goal of the journal is to serve the international scientific community in theoretical and mathematical physics by collecting and publishing original research papers meeting the highest professional standards in the field. The emphasis will be on analytical theoretical and mathematical physics in a broad sense.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


