Total Torsion and Spherical Curves Bending

IF 1.1 3区数学 Q1 MATHEMATICS

Mediterranean Journal of Mathematics Pub Date : 2024-03-20 DOI:10.1007/s00009-024-02595-3

Marija S. Najdanović, Svetozar R. Rančić, Ljubica S. Velimirović

引用次数: 0

Abstract

It is well known that the total torsion of a closed spherical curve is zero. Furthermore, if the total torsion of any closed curve on the surface is zero, then it is part of a plane or a sphere. In this paper, we examine the total torsion of a spherical curve during infinitesimal bending. We find the appropriate bending fields and show that the variation of the total torsion of a closed spherical curve is equal to zero. Some examples are considered both analytically and using our own software tool. For figures, we use colors to represent the value of torsion at different points of the curve, together with a colour-value scale.

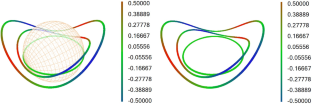
Abstract Image

查看原文本刊更多论文

总扭力和球形曲线弯曲

众所周知，封闭球面曲线的总扭力为零。此外，如果曲面上任何一条闭合曲线的总扭力为零，那么它就是平面或球面的一部分。本文研究了球形曲线在无限小弯曲过程中的总扭转。我们找到了适当的弯曲场，并证明闭合球形曲线的总扭力变化等于零。我们通过分析和使用自己的软件工具研究了一些例子。在图表中，我们使用颜色来表示曲线上不同点的扭力值以及色值刻度。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Mediterranean Journal of Mathematics 数学-数学

CiteScore

1.80

自引率

0.00%

发文量

261

审稿时长

6-12 weeks

期刊介绍： The Mediterranean Journal of Mathematics (MedJM) is a publication issued by the Department of Mathematics of the University of Bari. The new journal replaces the Conferenze del Seminario di Matematica dell’Università di Bari which has been in publication from 1954 until 2003. The Mediterranean Journal of Mathematics aims to publish original and high-quality peer-reviewed papers containing significant results across all fields of mathematics. The submitted papers should be of medium length (not to exceed 20 printed pages), well-written and appealing to a broad mathematical audience. In particular, the Mediterranean Journal of Mathematics intends to offer mathematicians from the Mediterranean countries a particular opportunity to circulate the results of their researches in a common journal. Through such a new cultural and scientific stimulus the journal aims to contribute to further integration amongst Mediterranean universities, though it is open to contribution from mathematicians across the world.