The residual set dimension of a generalized apollonian packing
IF 0.5
4区 数学
Q3 MATHEMATICS
引用次数: 0
Abstract
We view space-filling circle packings as subsets of the boundary of hyperbolic space subject to symmetry conditions based on a discrete group of isometries. This allows for the application of counting methods which admit rigorous upper and lower bounds on the Hausdorff dimension of the residual set of a generalized Apollonian circle packing. This dimension (which also coincides with a critical exponent) is strictly greater than that of the Apollonian packing.
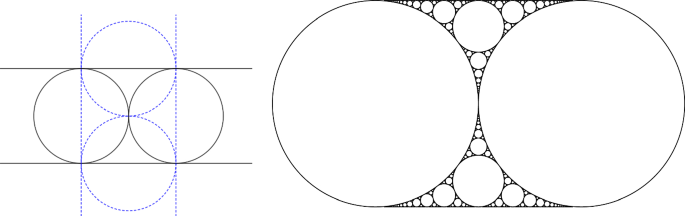
广义阿波罗包装的残集维度
我们将空间填充圆包装视为双曲空间边界的子集,受基于离散等距群的对称条件的制约。这样就可以应用计数方法,对广义阿波罗圆填料残余集的豪斯多夫维度进行严格的上下限计算。这个维度(也与临界指数重合)严格大于阿波罗圆包装的维度。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Geometriae Dedicata
数学-数学
CiteScore
0.90
自引率
0.00%
发文量
78
审稿时长
4-8 weeks
期刊介绍:
Geometriae Dedicata concentrates on geometry and its relationship to topology, group theory and the theory of dynamical systems.
Geometriae Dedicata aims to be a vehicle for excellent publications in geometry and related areas. Features of the journal will include:
A fast turn-around time for articles.
Special issues centered on specific topics.
All submitted papers should include some explanation of the context of the main results.
Geometriae Dedicata was founded in 1972 on the initiative of Hans Freudenthal in Utrecht, the Netherlands, who viewed geometry as a method rather than as a field. The present Board of Editors tries to continue in this spirit. The steady growth of the journal since its foundation is witness to the validity of the founder''s vision and to the success of the Editors'' mission.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


