Fractional commensurate model on COVID-19 with microbial co-infection: An optimal control analysis
G. M. Vijayalakshmi, P. Roselyn Besi, Ali Akgül
{"title":"Fractional commensurate model on COVID-19 with microbial co-infection: An optimal control analysis","authors":"G. M. Vijayalakshmi, P. Roselyn Besi, Ali Akgül","doi":"10.1002/oca.3093","DOIUrl":null,"url":null,"abstract":"Crossover behaviors have always existed in the history of infectious pandemics due to a few distinct, erratic spread outlines. This research aims to investigate the crossover behavior of the proposed SVICR commensurate fractional model for the COVID-19 delta variant, considering microbial coinfections. A mathematical model in terms of Atangana–Baleanu Caputo (ABC) category fractional integrals takes into account the co-infection of mucormycosis in immunocompromised COVID-19 patients caused by microbial infections. ABC operators preserve the intact history of the happenings under contemplation through its nonsingular kernel. It is observed that the framed five-compartmental SVICR model is positively bounded on R<sup>5</sup>, the solution space. Two equilibrium points <mjx-container aria-label=\"Menu available. Press control and space , or space\" ctxtmenu_counter=\"0\" ctxtmenu_oldtabindex=\"1\" jax=\"CHTML\" role=\"application\" sre-explorer- style=\"font-size: 103%; position: relative;\" tabindex=\"0\"><mjx-math aria-hidden=\"true\" location=\"graphic/oca3093-math-0001.png\"><mjx-semantics><mjx-mrow data-semantic-children=\"2,3,7\" data-semantic-collapsed=\"(10 (c 8 9) 2 3 7)\" data-semantic- data-semantic-role=\"text\" data-semantic-speech=\"upper E 0 and upper E Subscript e Baseline\" data-semantic-type=\"punctuated\"><mjx-msub data-semantic-children=\"0,1\" data-semantic- data-semantic-parent=\"10\" data-semantic-role=\"latinletter\" data-semantic-type=\"subscript\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"italic\" data-semantic- data-semantic-parent=\"2\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-script style=\"vertical-align: -0.15em; margin-left: -0.026em;\"><mjx-mn data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"2\" data-semantic-role=\"integer\" data-semantic-type=\"number\" size=\"s\"><mjx-c></mjx-c></mjx-mn></mjx-script></mjx-msub><mjx-mtext data-semantic-annotation=\"clearspeak:unit\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"10\" data-semantic-role=\"unknown\" data-semantic-type=\"text\"><mjx-c></mjx-c><mjx-c></mjx-c><mjx-c></mjx-c></mjx-mtext><mjx-mspace style=\"width: 0.25em;\"></mjx-mspace><mjx-msub data-semantic-children=\"5,6\" data-semantic- data-semantic-parent=\"10\" data-semantic-role=\"latinletter\" data-semantic-type=\"subscript\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"italic\" data-semantic- data-semantic-parent=\"7\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-script style=\"vertical-align: -0.15em; margin-left: -0.026em;\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"italic\" data-semantic- data-semantic-parent=\"7\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\" size=\"s\"><mjx-c></mjx-c></mjx-mi></mjx-script></mjx-msub></mjx-mrow></mjx-semantics></mjx-math><mjx-assistive-mml aria-hidden=\"true\" display=\"inline\" unselectable=\"on\"><math altimg=\"urn:x-wiley:01432087:media:oca3093:oca3093-math-0001\" display=\"inline\" location=\"graphic/oca3093-math-0001.png\" overflow=\"scroll\" xmlns=\"http://www.w3.org/1998/Math/MathML\"><semantics><mrow data-semantic-=\"\" data-semantic-children=\"2,3,7\" data-semantic-collapsed=\"(10 (c 8 9) 2 3 7)\" data-semantic-role=\"text\" data-semantic-speech=\"upper E 0 and upper E Subscript e Baseline\" data-semantic-type=\"punctuated\"><msub data-semantic-=\"\" data-semantic-children=\"0,1\" data-semantic-parent=\"10\" data-semantic-role=\"latinletter\" data-semantic-type=\"subscript\"><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"italic\" data-semantic-parent=\"2\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\">E</mi><mn data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-parent=\"2\" data-semantic-role=\"integer\" data-semantic-type=\"number\">0</mn></msub><mtext data-semantic-=\"\" data-semantic-annotation=\"clearspeak:unit\" data-semantic-font=\"normal\" data-semantic-parent=\"10\" data-semantic-role=\"unknown\" data-semantic-type=\"text\">and</mtext><mspace width=\"0.25em\"></mspace><msub data-semantic-=\"\" data-semantic-children=\"5,6\" data-semantic-parent=\"10\" data-semantic-role=\"latinletter\" data-semantic-type=\"subscript\"><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"italic\" data-semantic-parent=\"7\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\">E</mi><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"italic\" data-semantic-parent=\"7\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\">e</mi></msub></mrow>$$ {E}_0\\mathrm{and}\\ {E}_e $$</annotation></semantics></math></mjx-assistive-mml></mjx-container> representing the survival and annihilation of sickness respectively are contributed by the single population N(t), which is counted in five dependent compartments: <mjx-container aria-label=\"Menu available. Press control and space , or space\" ctxtmenu_counter=\"1\" ctxtmenu_oldtabindex=\"1\" jax=\"CHTML\" role=\"application\" sre-explorer- style=\"font-size: 103%; position: relative;\" tabindex=\"0\"><mjx-math aria-hidden=\"true\" location=\"graphic/oca3093-math-0002.png\"><mjx-semantics><mjx-mrow data-semantic-children=\"33,5,35,11,37,17,39,23,43,31\" data-semantic-content=\"5,11,17,23,31\" data-semantic- data-semantic-role=\"sequence\" data-semantic-speech=\"normal upper S left parenthesis normal t right parenthesis comma normal upper V left parenthesis normal t right parenthesis comma normal upper I left parenthesis normal t right parenthesis comma normal upper C left parenthesis normal t right parenthesis comma and normal upper R left parenthesis normal t right parenthesis period\" data-semantic-type=\"punctuated\"><mjx-mrow data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"0,4\" data-semantic-content=\"32,0\" data-semantic- data-semantic-parent=\"44\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"33\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic-added=\"true\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"33\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-children=\"2\" data-semantic-content=\"1,3\" data-semantic- data-semantic-parent=\"33\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"4\" data-semantic-role=\"open\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"4\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"4\" data-semantic-role=\"close\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo></mjx-mrow></mjx-mrow><mjx-mo data-semantic- data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"comma\" data-semantic-type=\"punctuation\" rspace=\"3\" style=\"margin-left: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"6,10\" data-semantic-content=\"34,6\" data-semantic- data-semantic-parent=\"44\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"35\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic-added=\"true\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"35\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-children=\"8\" data-semantic-content=\"7,9\" data-semantic- data-semantic-parent=\"35\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"10\" data-semantic-role=\"open\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"10\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"10\" data-semantic-role=\"close\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo></mjx-mrow></mjx-mrow><mjx-mo data-semantic- data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"comma\" data-semantic-type=\"punctuation\" rspace=\"3\" style=\"margin-left: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"12,16\" data-semantic-content=\"36,12\" data-semantic- data-semantic-parent=\"44\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"37\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic-added=\"true\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"37\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-children=\"14\" data-semantic-content=\"13,15\" data-semantic- data-semantic-parent=\"37\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"16\" data-semantic-role=\"open\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"16\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"16\" data-semantic-role=\"close\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo></mjx-mrow></mjx-mrow><mjx-mo data-semantic- data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"comma\" data-semantic-type=\"punctuation\" rspace=\"3\" style=\"margin-left: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"18,22\" data-semantic-content=\"38,18\" data-semantic- data-semantic-parent=\"44\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"39\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic-added=\"true\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"39\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-children=\"20\" data-semantic-content=\"19,21\" data-semantic- data-semantic-parent=\"39\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"22\" data-semantic-role=\"open\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"22\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"22\" data-semantic-role=\"close\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo></mjx-mrow></mjx-mrow><mjx-mo data-semantic- data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"comma\" data-semantic-type=\"punctuation\" rspace=\"3\" style=\"margin-left: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-children=\"24,41\" data-semantic-collapsed=\"(43 (c 42) 24 41)\" data-semantic- data-semantic-parent=\"44\" data-semantic-role=\"text\" data-semantic-type=\"punctuated\"><mjx-mtext data-semantic-annotation=\"clearspeak:unit\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"43\" data-semantic-role=\"unknown\" data-semantic-type=\"text\"><mjx-c></mjx-c><mjx-c></mjx-c><mjx-c></mjx-c></mjx-mtext><mjx-mspace style=\"width: 0.25em;\"></mjx-mspace><mjx-mrow data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"26,30\" data-semantic-content=\"40,26\" data-semantic- data-semantic-parent=\"43\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"41\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic-added=\"true\" data-semantic- data-semantic-operator=\"appl\" data-semantic-parent=\"41\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mrow data-semantic-children=\"28\" data-semantic-content=\"27,29\" data-semantic- data-semantic-parent=\"41\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"30\" data-semantic-role=\"open\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"30\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-mo data-semantic- data-semantic-operator=\"fenced\" data-semantic-parent=\"30\" data-semantic-role=\"close\" data-semantic-type=\"fence\" style=\"margin-left: 0.056em; margin-right: 0.056em;\"><mjx-c></mjx-c></mjx-mo></mjx-mrow></mjx-mrow></mjx-mrow><mjx-mo data-semantic- data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"fullstop\" data-semantic-type=\"punctuation\" rspace=\"3\" style=\"margin-left: 0.056em;\"><mjx-c></mjx-c></mjx-mo></mjx-mrow></mjx-semantics></mjx-math><mjx-assistive-mml aria-hidden=\"true\" display=\"inline\" unselectable=\"on\"><math altimg=\"urn:x-wiley:01432087:media:oca3093:oca3093-math-0002\" display=\"inline\" location=\"graphic/oca3093-math-0002.png\" overflow=\"scroll\" xmlns=\"http://www.w3.org/1998/Math/MathML\"><semantics><mrow data-semantic-=\"\" data-semantic-children=\"33,5,35,11,37,17,39,23,43,31\" data-semantic-content=\"5,11,17,23,31\" data-semantic-role=\"sequence\" data-semantic-speech=\"normal upper S left parenthesis normal t right parenthesis comma normal upper V left parenthesis normal t right parenthesis comma normal upper I left parenthesis normal t right parenthesis comma normal upper C left parenthesis normal t right parenthesis comma and normal upper R left parenthesis normal t right parenthesis period\" data-semantic-type=\"punctuated\"><mrow data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"0,4\" data-semantic-content=\"32,0\" data-semantic-parent=\"44\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-operator=\"appl\" data-semantic-parent=\"33\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\" mathvariant=\"normal\">S</mi><mo data-semantic-=\"\" data-semantic-added=\"true\" data-semantic-operator=\"appl\" data-semantic-parent=\"33\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\"></mo><mrow data-semantic-=\"\" data-semantic-children=\"2\" data-semantic-content=\"1,3\" data-semantic-parent=\"33\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"4\" data-semantic-role=\"open\" data-semantic-type=\"fence\" stretchy=\"false\">(</mo><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-parent=\"4\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\" mathvariant=\"normal\">t</mi><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"4\" data-semantic-role=\"close\" data-semantic-type=\"fence\" stretchy=\"false\">)</mo></mrow></mrow><mo data-semantic-=\"\" data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"comma\" data-semantic-type=\"punctuation\">,</mo><mrow data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"6,10\" data-semantic-content=\"34,6\" data-semantic-parent=\"44\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-operator=\"appl\" data-semantic-parent=\"35\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\" mathvariant=\"normal\">V</mi><mo data-semantic-=\"\" data-semantic-added=\"true\" data-semantic-operator=\"appl\" data-semantic-parent=\"35\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\"></mo><mrow data-semantic-=\"\" data-semantic-children=\"8\" data-semantic-content=\"7,9\" data-semantic-parent=\"35\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"10\" data-semantic-role=\"open\" data-semantic-type=\"fence\" stretchy=\"false\">(</mo><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-parent=\"10\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\" mathvariant=\"normal\">t</mi><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"10\" data-semantic-role=\"close\" data-semantic-type=\"fence\" stretchy=\"false\">)</mo></mrow></mrow><mo data-semantic-=\"\" data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"comma\" data-semantic-type=\"punctuation\">,</mo><mrow data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"12,16\" data-semantic-content=\"36,12\" data-semantic-parent=\"44\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-operator=\"appl\" data-semantic-parent=\"37\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\" mathvariant=\"normal\">I</mi><mo data-semantic-=\"\" data-semantic-added=\"true\" data-semantic-operator=\"appl\" data-semantic-parent=\"37\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\"></mo><mrow data-semantic-=\"\" data-semantic-children=\"14\" data-semantic-content=\"13,15\" data-semantic-parent=\"37\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"16\" data-semantic-role=\"open\" data-semantic-type=\"fence\" stretchy=\"false\">(</mo><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-parent=\"16\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\" mathvariant=\"normal\">t</mi><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"16\" data-semantic-role=\"close\" data-semantic-type=\"fence\" stretchy=\"false\">)</mo></mrow></mrow><mo data-semantic-=\"\" data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"comma\" data-semantic-type=\"punctuation\">,</mo><mrow data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"18,22\" data-semantic-content=\"38,18\" data-semantic-parent=\"44\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-operator=\"appl\" data-semantic-parent=\"39\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\" mathvariant=\"normal\">C</mi><mo data-semantic-=\"\" data-semantic-added=\"true\" data-semantic-operator=\"appl\" data-semantic-parent=\"39\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\"></mo><mrow data-semantic-=\"\" data-semantic-children=\"20\" data-semantic-content=\"19,21\" data-semantic-parent=\"39\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"22\" data-semantic-role=\"open\" data-semantic-type=\"fence\" stretchy=\"false\">(</mo><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-parent=\"22\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\" mathvariant=\"normal\">t</mi><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"22\" data-semantic-role=\"close\" data-semantic-type=\"fence\" stretchy=\"false\">)</mo></mrow></mrow><mo data-semantic-=\"\" data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"comma\" data-semantic-type=\"punctuation\">,</mo><mrow data-semantic-=\"\" data-semantic-children=\"24,41\" data-semantic-collapsed=\"(43 (c 42) 24 41)\" data-semantic-parent=\"44\" data-semantic-role=\"text\" data-semantic-type=\"punctuated\"><mtext data-semantic-=\"\" data-semantic-annotation=\"clearspeak:unit\" data-semantic-font=\"normal\" data-semantic-parent=\"43\" data-semantic-role=\"unknown\" data-semantic-type=\"text\">and</mtext><mspace width=\"0.25em\"></mspace><mrow data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-children=\"26,30\" data-semantic-content=\"40,26\" data-semantic-parent=\"43\" data-semantic-role=\"simple function\" data-semantic-type=\"appl\"><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-operator=\"appl\" data-semantic-parent=\"41\" data-semantic-role=\"simple function\" data-semantic-type=\"identifier\" mathvariant=\"normal\">R</mi><mo data-semantic-=\"\" data-semantic-added=\"true\" data-semantic-operator=\"appl\" data-semantic-parent=\"41\" data-semantic-role=\"application\" data-semantic-type=\"punctuation\"></mo><mrow data-semantic-=\"\" data-semantic-children=\"28\" data-semantic-content=\"27,29\" data-semantic-parent=\"41\" data-semantic-role=\"leftright\" data-semantic-type=\"fenced\"><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"30\" data-semantic-role=\"open\" data-semantic-type=\"fence\" stretchy=\"false\">(</mo><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-parent=\"30\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\" mathvariant=\"normal\">t</mi><mo data-semantic-=\"\" data-semantic-operator=\"fenced\" data-semantic-parent=\"30\" data-semantic-role=\"close\" data-semantic-type=\"fence\" stretchy=\"false\">)</mo></mrow></mrow></mrow><mo data-semantic-=\"\" data-semantic-operator=\"punctuated\" data-semantic-parent=\"44\" data-semantic-role=\"fullstop\" data-semantic-type=\"punctuation\">.</mo></mrow>$$ \\mathrm{S}\\left(\\mathrm{t}\\right),\\mathrm{V}\\left(\\mathrm{t}\\right),\\mathrm{I}\\left(\\mathrm{t}\\right),\\mathrm{C}\\left(\\mathrm{t}\\right),\\mathrm{and}\\ \\mathrm{R}\\left(\\mathrm{t}\\right). $$</annotation></semantics></math></mjx-assistive-mml></mjx-container> The bilinear growth rate of new additional infections from the contagious infectives over time ‘t’ is viewed through the threshold metric <mjx-container aria-label=\"Menu available. Press control and space , or space\" ctxtmenu_counter=\"2\" ctxtmenu_oldtabindex=\"1\" jax=\"CHTML\" role=\"application\" sre-explorer- style=\"font-size: 103%; position: relative;\" tabindex=\"0\"><mjx-math aria-hidden=\"true\" location=\"graphic/oca3093-math-0003.png\"><mjx-semantics><mjx-mrow><mjx-mspace style=\"width: 0.25em;\"></mjx-mspace><mjx-mrow data-semantic-children=\"3,4\" data-semantic-content=\"4\" data-semantic- data-semantic-role=\"endpunct\" data-semantic-speech=\"upper R 0 period\" data-semantic-type=\"punctuated\"><mjx-msub data-semantic-children=\"1,2\" data-semantic- data-semantic-parent=\"5\" data-semantic-role=\"latinletter\" data-semantic-type=\"subscript\"><mjx-mi data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"italic\" data-semantic- data-semantic-parent=\"3\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\"><mjx-c></mjx-c></mjx-mi><mjx-script style=\"vertical-align: -0.15em;\"><mjx-mn data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic- data-semantic-parent=\"3\" data-semantic-role=\"integer\" data-semantic-type=\"number\" size=\"s\"><mjx-c></mjx-c></mjx-mn></mjx-script></mjx-msub><mjx-mo data-semantic- data-semantic-operator=\"punctuated\" data-semantic-parent=\"5\" data-semantic-role=\"fullstop\" data-semantic-type=\"punctuation\" rspace=\"3\" style=\"margin-left: 0.056em;\"><mjx-c></mjx-c></mjx-mo></mjx-mrow></mjx-mrow></mjx-semantics></mjx-math><mjx-assistive-mml display=\"inline\" unselectable=\"on\"><math altimg=\"urn:x-wiley:01432087:media:oca3093:oca3093-math-0003\" display=\"inline\" location=\"graphic/oca3093-math-0003.png\" overflow=\"scroll\" xmlns=\"http://www.w3.org/1998/Math/MathML\"><semantics><mrow><mspace width=\"0.25em\"></mspace><mrow data-semantic-=\"\" data-semantic-children=\"3,4\" data-semantic-content=\"4\" data-semantic-role=\"endpunct\" data-semantic-speech=\"upper R 0 period\" data-semantic-type=\"punctuated\"><msub data-semantic-=\"\" data-semantic-children=\"1,2\" data-semantic-parent=\"5\" data-semantic-role=\"latinletter\" data-semantic-type=\"subscript\"><mi data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"italic\" data-semantic-parent=\"3\" data-semantic-role=\"latinletter\" data-semantic-type=\"identifier\">R</mi><mn data-semantic-=\"\" data-semantic-annotation=\"clearspeak:simple\" data-semantic-font=\"normal\" data-semantic-parent=\"3\" data-semantic-role=\"integer\" data-semantic-type=\"number\">0</mn></msub><mo data-semantic-=\"\" data-semantic-operator=\"punctuated\" data-semantic-parent=\"5\" data-semantic-role=\"fullstop\" data-semantic-type=\"punctuation\">.</mo></mrow></mrow>$$ {R}_0. $$</annotation></semantics></math></mjx-assistive-mml></mjx-container> Lyapunov's stability function examines the parametric influences over the virulent spread globally. The significant focus is to investigate the Mucormycosis cases in COVID-19 patients with underlying diabetic complications. Diabetes mellitus is the major concern for several coinfections among COVID-19 recoveries. Aiming to minimalize the critical states, an Lagrangian–Hamiltonian optimum control structure is also performed for the SVICR model by introducing control variables in effect to tri-control probes of minimized contact rates, persuasive vaccinations, and glycemic control of post recovered diabetic patients. The hike in the Severity of the ailment due to fungal pathogens is studied through numerical convergence of predictor–corrector scheme and simulations. Using estimated parametric values from the statistical data of mucormycosis and infections of COVID-19 reported cases in India, the prominence of control effects are visualized graphically. To conclude, a complete qualitative analysis of the minimization problem is executed for different levels of control values. We avow that effective control intrusions would almost certainly decline the complexities associated with the viral pathogens.","PeriodicalId":501055,"journal":{"name":"Optimal Control Applications and Methods","volume":"39 1","pages":""},"PeriodicalIF":0.0000,"publicationDate":"2024-01-08","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":null,"platform":"Semanticscholar","paperid":null,"PeriodicalName":"Optimal Control Applications and Methods","FirstCategoryId":"1085","ListUrlMain":"https://doi.org/10.1002/oca.3093","RegionNum":0,"RegionCategory":null,"ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"","JCRName":"","Score":null,"Total":0}
引用次数: 0
Abstract
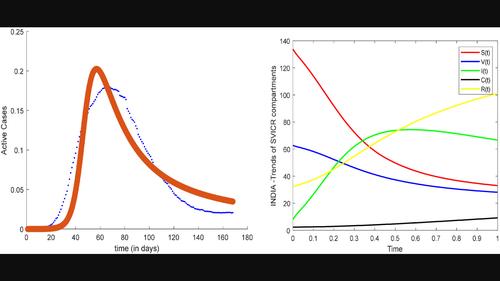
Crossover behaviors have always existed in the history of infectious pandemics due to a few distinct, erratic spread outlines. This research aims to investigate the crossover behavior of the proposed SVICR commensurate fractional model for the COVID-19 delta variant, considering microbial coinfections. A mathematical model in terms of Atangana–Baleanu Caputo (ABC) category fractional integrals takes into account the co-infection of mucormycosis in immunocompromised COVID-19 patients caused by microbial infections. ABC operators preserve the intact history of the happenings under contemplation through its nonsingular kernel. It is observed that the framed five-compartmental SVICR model is positively bounded on R5, the solution space. Two equilibrium points
COVID-19 微生物共感染的分数相称模型:最优控制分析
在传染病大流行的历史上,由于一些不同的、不规则的传播轮廓,交叉行为一直存在。本研究旨在研究针对 COVID-19 delta 变种提出的 SVICR 相称分数模型的交叉行为,同时考虑到微生物的共感染。阿坦加纳-巴莱亚努-卡普托(Atangana-Baleanu Caputo,ABC)类分数积分数学模型考虑了由微生物感染引起的免疫力低下的 COVID-19 患者的粘液瘤病合并感染。ABC 算子通过其非奇异内核保留了所考虑事件的完整历史。据观察,框架五室 SVICR 模型在解空间 R5 上是正约束的。两个平衡点 E0 和 Ee$$ {E}_0\mathrm{and}\ {E}_e$$ 分别代表疾病的存活和消灭,它们由单一种群 N(t) 贡献,N(t) 被计入五个从属区室:S(t)、V(t)、I(t)、C(t)和R(t)。$$ \mathrm{S}\left(\mathrm{t}\right),\mathrm{V}\left(\mathrm{t}\right),\mathrm{I}\left(\mathrm{t}\right),\mathrm{C}\left(\mathrm{t}\right),\mathrm{and}\mathrm{R}\left(\mathrm{t}\right).$$ 通过阈值度量 R0.$$ {R}_0,可以看到在 "t "时间内传染性感染者新增感染的双线性增长率。$$ Lyapunov 稳定函数考察了对全球病毒传播的参数影响。重点是调查 COVID-19 患者中伴有潜在糖尿病并发症的粘孢子菌病例。在 COVID-19 的康复者中,糖尿病是几种合并感染的主要问题。为了将临界状态最小化,我们还通过引入控制变量,对 SVICR 模型进行了拉格朗日-哈密尔顿最优控制结构分析,以实现最小化接触率、说服性疫苗接种和康复后糖尿病患者血糖控制的三重控制。通过预测-校正方案的数值收敛和模拟,研究了真菌病原体导致的疾病严重程度的增加。利用印度 COVID-19 报告病例中粘孢子菌病和感染统计数据的估计参数值,以图形方式直观显示了控制效果的显著性。最后,针对不同的控制值水平,对最小化问题进行了完整的定性分析。我们认为,有效的控制入侵几乎肯定会降低与病毒病原体相关的复杂性。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
相关文献
×
引用
GB/T 7714-2015
复制
MLA
复制
APA
复制
导出至
BibTeX
EndNote
RefMan
NoteFirst
NoteExpress
请完成安全验证×
微信好友
朋友圈
QQ好友
复制链接
取消

已复制链接
快去分享给好友吧!
我知道了

点击右上角分享

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:
