Application of the Local Discontinuous Galerkin Method to the Solution of the Quasi-Gas Dynamic System of Equations

Q3 Mathematics

Mathematical Models and Computer Simulations Pub Date : 2023-12-26 DOI:10.1134/s207004822307013x

E. V. Shilnikov, I. R. Khaytaliev

引用次数: 0

Abstract

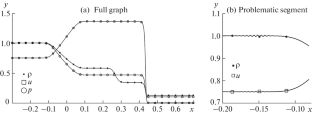
The solution of a quasi-gas dynamic (QGD) system of equations using the local discontinuous Galerkin method (LDG) is considered. One-dimensional Riemann discontinuity problems with known exact solutions are solved. Strong discontinuities are present in the solutions of the problems. Therefore, to ensure the monotonicity of the solution obtained by the LDG method, the so-called slope limiters, or limiters, are introduced. A “moment” limiter is chosen that preserves as high an order as possible. The limiter is modified to smooth the oscillations in the areas where the solution is constant.

Abstract Image

查看原文本刊更多论文

应用局部非连续伽勒金方法求解准气体动态方程组

摘要研究了利用局部不连续伽勒金方法（LDG）求解准气体动力学（QGD）方程组的问题。求解了已知精确解的一维黎曼不连续问题。问题的解中存在强不连续性。因此，为了确保 LDG 方法求解的单调性，引入了所谓的斜率限制器或限制器。我们选择了一个 "矩 "限制器，以尽可能保留高阶。对限制器进行修改，以平滑解恒定区域的振荡。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Mathematical Models and Computer Simulations Mathematics-Computational Mathematics

CiteScore

1.20

自引率

0.00%

发文量

期刊介绍： Mathematical Models and Computer Simulations is a journal that publishes high-quality and original articles at the forefront of development of mathematical models, numerical methods, computer-assisted studies in science and engineering with the potential for impact across the sciences, and construction of massively parallel codes for supercomputers. The problem-oriented papers are devoted to various problems including industrial mathematics, numerical simulation in multiscale and multiphysics, materials science, chemistry, economics, social, and life sciences.