Correcting spot power variation estimator via Edgeworth expansion

Abstract

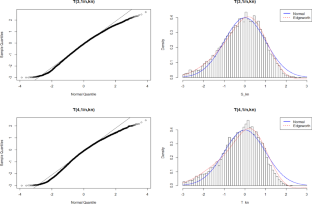
In this paper, we propose an estimator of power spot volatility of order p through Edgeworth expansion. We provide a precise description of how to compute the expansion and the first four cumulants are given in an explicit form. We also construct feasible confidence intervals (one-sided and two-sided) for the pth power spot volatility estimator by using Edgeworth expansion. A Monte Carlo simulation study shows that the confidence intervals and probability density curve based on Edgeworth expansion perform better than the conventional case based on Normal approximation.