On a nonlinear elasticity problem with friction and Sobolev spaces with variable exponents

Fixed Point Theory and Applications Pub Date : 2022-06-01 DOI:10.1186/s13663-022-00724-9

Boukrouche, Mahdi, Merouani, Boubakeur, Zoubai, Fayrouz

引用次数: 0

Abstract

We consider a nonlinear elasticity problem in a bounded domain, its boundary is decomposed in three parts: lower, upper, and lateral. The displacement of the substance, which is the unknown of the problem, is assumed to satisfy the homogeneous Dirichlet boundary conditions on the upper part, and not homogeneous one on the lateral part, while on the lower part, friction conditions are considered. In addition, the problem is governed by a particular constitutive law of elasticity system with a strongly nonlinear strain tensor. The functional framework leads to using Sobolev spaces with variable exponents. The formulation of the problem leads to a variational inequality, for which we prove the existence and uniqueness of the solution of the associated variational problem.

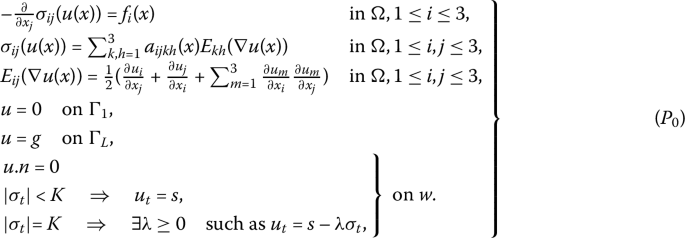
Abstract Image

查看原文本刊更多论文

具有摩擦和变指数Sobolev空间的非线性弹性问题

考虑有界域上的非线性弹性问题，将其边界分解为下、上、侧三部分。问题中未知的物质位移在上半部分满足齐次Dirichlet边界条件，而在侧边部分不满足齐次Dirichlet边界条件，下半部分考虑摩擦条件。此外，该问题受弹性系统具有强非线性应变张量的特定本构律支配。函数框架导致使用可变指数的Sobolev空间。该问题的形式导致了一个变分不等式，为此我们证明了相关变分问题解的存在唯一性。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Fixed Point Theory and Applications MATHEMATICS, APPLIED-MATHEMATICS

自引率

0.00%

发文量

期刊介绍： In a wide range of mathematical, computational, economical, modeling and engineering problems, the existence of a solution to a theoretical or real world problem is equivalent to the existence of a fixed point for a suitable map or operator. Fixed points are therefore of paramount importance in many areas of mathematics, sciences and engineering. The theory itself is a beautiful mixture of analysis (pure and applied), topology and geometry. Over the last 60 years or so, the theory of fixed points has been revealed as a very powerful and important tool in the study of nonlinear phenomena. In particular, fixed point techniques have been applied in such diverse fields as biology, chemistry, physics, engineering, game theory and economics. In numerous cases finding the exact solution is not possible; hence it is necessary to develop appropriate algorithms to approximate the requested result. This is strongly related to control and optimization problems arising in the different sciences and in engineering problems. Many situations in the study of nonlinear equations, calculus of variations, partial differential equations, optimal control and inverse problems can be formulated in terms of fixed point problems or optimization.