A polynomial interior-point algorithm with improved iteration bounds for linear optimization

Abstract

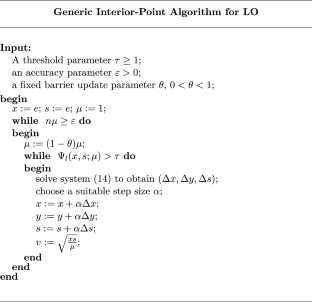
In this paper, we present a polynomial primal-dual interior-point algorithm for linear optimization based on a modified logarithmic barrier kernel function. Iteration bounds for the large-update interior-point method and the small-update interior-point method are derived. It is shown that the large-update interior-point method has the same polynomial complexity as the small-update interior-point method, which is the best known iteration bounds. Our result closes a long-existing gap in the theoretical complexity bounds for large-update interior-point method and small-update interior-point method.