Deterministic n-person shortest path and terminal games on symmetric digraphs have Nash equilibria in pure stationary strategies
IF 0.4
4区 经济学
Q4 ECONOMICS
引用次数: 2
Abstract
Abstract We prove that a deterministic n -person shortest path game has a Nash equlibrium in pure and stationary strategies if it is edge-symmetric (that is ( u , v ) is a move whenever ( v , u ) is, apart from moves entering terminal vertices) and the length of every move is positive for each player. Both conditions are essential, though it remains an open problem whether there exists a NE-free 2-person non-edge-symmetric game with positive lengths. We provide examples for NE-free 2-person edge-symmetric games that are not positive. We also consider the special case of terminal games (shortest path games in which only terminal moves have nonzero length, possibly negative) and prove that edge-symmetric n -person terminal games always have Nash equilibria in pure and stationary strategies. Furthermore, we prove that an edge-symmetric 2-person terminal game has a uniform (subgame perfect) Nash equilibrium, provided any infinite play is worse than any of the terminals for both players.
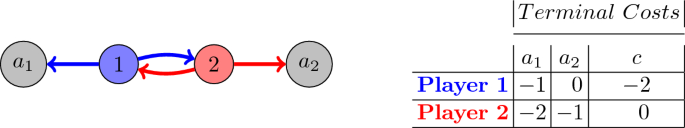
对称有向图上的确定性n人最短路径和终端对策在纯平稳策略下具有纳什均衡
摘要我们证明了一个确定性n人最短路径博弈在纯平稳策略下具有纳什均衡,如果它是边对称的(即(u, v)是一个移动,当(v, u)为时,除了移动进入终端顶点外),并且每个参与者的每一步移动的长度都是正的。这两个条件都是必要的,尽管是否存在一个长度为正的无ne的2人非边对称博弈仍然是一个悬而未决的问题。我们提供了非正的无ne的2人边对称博弈的例子。我们还考虑了终端博弈的特殊情况(在最短路径博弈中,只有终端的移动是非零长度的,可能是负的),并证明了边对称的n人终端博弈在纯平稳策略下总是具有纳什均衡。进一步,我们证明了一个边对称的2人终端博弈具有统一的(子博弈完美的)纳什均衡,假设任何无限的玩法都比两个玩家的任何终端都差。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

International Journal of Game Theory
数学-数学跨学科应用
CiteScore
1.30
自引率
0.00%
发文量
9
审稿时长
1 months
期刊介绍:
International Journal of Game Theory is devoted to game theory and its applications. It publishes original research making significant contributions from a methodological, conceptual or mathematical point of view. Survey articles may also be considered if especially useful for the field.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


