Cat(0) polygonal complexes are 2-median
IF 0.5
4区 数学
Q3 MATHEMATICS
引用次数: 0
Abstract
Abstract Median spaces are spaces in which for every three points the three intervals between them intersect at a single point. It is well known that rank-1 affine buildings are median spaces, but by a result of Haettel, higher rank buildings are not even coarse median. We define the notion of “2-median space”, which roughly says that for every four points the minimal discs filling the four geodesic triangles they span intersect in a point or a geodesic segment. We show that CAT(0) Euclidean polygonal complexes, and in particular rank-2 affine buildings, are 2-median. In the appendix, we recover a special case of a result of Stadler of a Fary–Milnor type theorem and show in elementary tools that a minimal disc filling a geodesic triangle is injective.
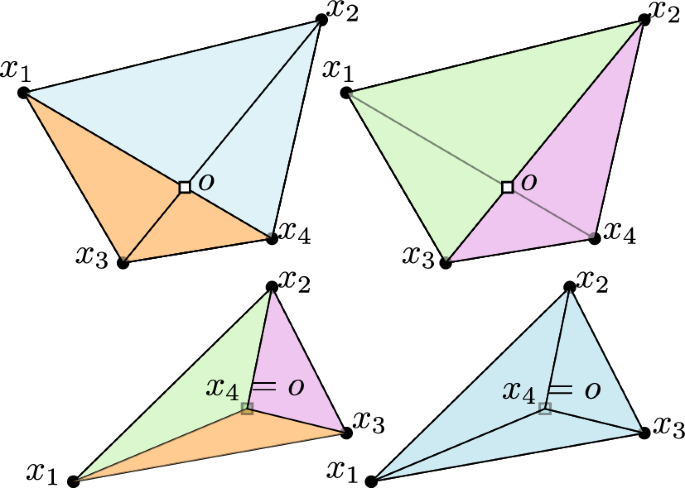
Cat(0)多边形配合物为2中位数
中位数空间是每三个点之间的三个间隔相交于一个点的空间。众所周知,1级仿射建筑是中位数空间,但由于Haettel,更高级别的建筑甚至不是粗中位数。我们定义了“2-中位数空间”的概念,粗略地说,对于每四个点,填充四个测地线三角形的最小圆盘相交于一个点或测地线段。我们证明了CAT(0)欧几里得多边形复合物,特别是2阶仿射建筑物,是2中位数的。在附录中,我们恢复了法里-米尔诺型定理的Stadler结果的一个特例,并在初等工具中证明了填充测地三角形的极小圆盘是内射的。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Geometriae Dedicata
数学-数学
CiteScore
0.90
自引率
0.00%
发文量
78
审稿时长
4-8 weeks
期刊介绍:
Geometriae Dedicata concentrates on geometry and its relationship to topology, group theory and the theory of dynamical systems.
Geometriae Dedicata aims to be a vehicle for excellent publications in geometry and related areas. Features of the journal will include:
A fast turn-around time for articles.
Special issues centered on specific topics.
All submitted papers should include some explanation of the context of the main results.
Geometriae Dedicata was founded in 1972 on the initiative of Hans Freudenthal in Utrecht, the Netherlands, who viewed geometry as a method rather than as a field. The present Board of Editors tries to continue in this spirit. The steady growth of the journal since its foundation is witness to the validity of the founder''s vision and to the success of the Editors'' mission.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


