Double-slit X-ray dynamical diffraction in curved crystals.

IF 1.9 4区材料科学 Q3 CHEMISTRY, MULTIDISCIPLINARY

Acta Crystallographica Section A: Foundations and Advances Pub Date : 2023-07-01 DOI:10.1107/S2053273323004345

Minas K Balyan

引用次数: 0

Abstract

The theoretical investigation of double-slit X-ray dynamical diffraction in curved crystals shows that Young's interference fringes are formed. An expression for the period of the fringes has been established which is polarization sensitive. The position of the fringes in the cross section of the beam depends on the deviation from the Bragg exact orientation for a perfect crystal, on the curvature radius and on the thickness of the crystal. This type of diffraction can be used for determination of the curvature radius by measuring the shift of the fringes from the centre of the beam.

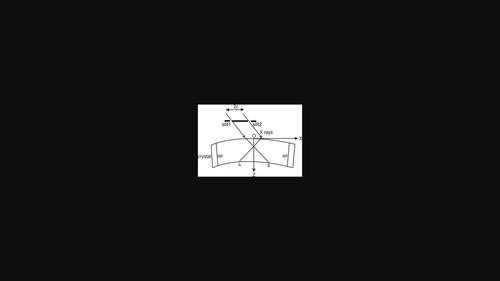
Abstract Image

查看原文本刊更多论文

弯曲晶体中的双缝x射线动态衍射。

对弯曲晶体中双缝x射线动态衍射的理论研究表明，存在杨氏干涉条纹。建立了偏振敏感的条纹周期表达式。条纹在光束横截面上的位置取决于完美晶体与布拉格精确方向的偏差、曲率半径和晶体的厚度。这种类型的衍射可以通过测量条纹从光束中心的位移来确定曲率半径。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Acta Crystallographica Section A: Foundations and Advances CHEMISTRY, MULTIDISCIPLINARYCRYSTALLOGRAPH-CRYSTALLOGRAPHY

CiteScore

2.60

自引率

11.10%

发文量

419

期刊介绍： Acta Crystallographica Section A: Foundations and Advances publishes articles reporting advances in the theory and practice of all areas of crystallography in the broadest sense. As well as traditional crystallography, this includes nanocrystals, metacrystals, amorphous materials, quasicrystals, synchrotron and XFEL studies, coherent scattering, diffraction imaging, time-resolved studies and the structure of strain and defects in materials. The journal has two parts, a rapid-publication Advances section and the traditional Foundations section. Articles for the Advances section are of particularly high value and impact. They receive expedited treatment and may be highlighted by an accompanying scientific commentary article and a press release. Further details are given in the November 2013 Editorial. The central themes of the journal are, on the one hand, experimental and theoretical studies of the properties and arrangements of atoms, ions and molecules in condensed matter, periodic, quasiperiodic or amorphous, ideal or real, and, on the other, the theoretical and experimental aspects of the various methods to determine these properties and arrangements.