非平凡束与代数经典场论

IF 1.4 3区物理与天体物理 Q2 PHYSICS, MATHEMATICAL

Annales Henri Poincaré Pub Date : 2023-11-22 DOI:10.1007/s00023-023-01386-y

Romeo Brunetti, Andrea Moro

{"title":"非平凡束与代数经典场论","authors":"Romeo Brunetti, Andrea Moro","doi":"10.1007/s00023-023-01386-y","DOIUrl":null,"url":null,"abstract":"<p>Inspired by the recent algebraic approach to classical field theory, we propose a more general setting based on the manifold of smooth sections of a non-trivial fiber bundle. Central is the notion of observables over such sections, i.e., appropriate smooth functions on them. The kinematics will be further specified by means of the Peierls brackets, which in turn are defined via the causal propagators of linearized field equations. We shall compare the formalism we use with the more traditional ones.</p>","PeriodicalId":463,"journal":{"name":"Annales Henri Poincaré","volume":"191 1","pages":""},"PeriodicalIF":1.4000,"publicationDate":"2023-11-22","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"Non-trivial Bundles and Algebraic Classical Field Theory\",\"authors\":\"Romeo Brunetti, Andrea Moro\",\"doi\":\"10.1007/s00023-023-01386-y\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<p>Inspired by the recent algebraic approach to classical field theory, we propose a more general setting based on the manifold of smooth sections of a non-trivial fiber bundle. Central is the notion of observables over such sections, i.e., appropriate smooth functions on them. The kinematics will be further specified by means of the Peierls brackets, which in turn are defined via the causal propagators of linearized field equations. We shall compare the formalism we use with the more traditional ones.</p>\",\"PeriodicalId\":463,\"journal\":{\"name\":\"Annales Henri Poincaré\",\"volume\":\"191 1\",\"pages\":\"\"},\"PeriodicalIF\":1.4000,\"publicationDate\":\"2023-11-22\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Annales Henri Poincaré\",\"FirstCategoryId\":\"4\",\"ListUrlMain\":\"https://doi.org/10.1007/s00023-023-01386-y\",\"RegionNum\":3,\"RegionCategory\":\"物理与天体物理\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q2\",\"JCRName\":\"PHYSICS, MATHEMATICAL\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Annales Henri Poincaré","FirstCategoryId":"4","ListUrlMain":"https://doi.org/10.1007/s00023-023-01386-y","RegionNum":3,"RegionCategory":"物理与天体物理","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q2","JCRName":"PHYSICS, MATHEMATICAL","Score":null,"Total":0}

引用次数: 0

摘要

受经典场论最近的代数方法的启发，我们提出了一个基于非平凡纤维束光滑截面流形的更一般的设置。核心是这些部分上的可观察对象的概念，即在它们上适当的平滑函数。运动学将通过佩尔斯括号进一步说明，而佩尔斯括号又通过线性化场方程的因果传播量来定义。我们将把我们使用的形式主义与比较传统的形式主义进行比较。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

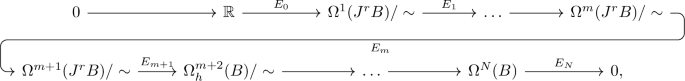
Non-trivial Bundles and Algebraic Classical Field Theory

查看原文本刊更多论文

Non-trivial Bundles and Algebraic Classical Field Theory

Inspired by the recent algebraic approach to classical field theory, we propose a more general setting based on the manifold of smooth sections of a non-trivial fiber bundle. Central is the notion of observables over such sections, i.e., appropriate smooth functions on them. The kinematics will be further specified by means of the Peierls brackets, which in turn are defined via the causal propagators of linearized field equations. We shall compare the formalism we use with the more traditional ones.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Annales Henri Poincaré 物理-物理：粒子与场物理

CiteScore

3.00

自引率

6.70%

发文量

108

审稿时长

6-12 weeks

期刊介绍： The two journals Annales de l''Institut Henri Poincaré, physique théorique and Helvetica Physical Acta merged into a single new journal under the name Annales Henri Poincaré - A Journal of Theoretical and Mathematical Physics edited jointly by the Institut Henri Poincaré and by the Swiss Physical Society. The goal of the journal is to serve the international scientific community in theoretical and mathematical physics by collecting and publishing original research papers meeting the highest professional standards in the field. The emphasis will be on analytical theoretical and mathematical physics in a broad sense.